Introduction to mathematical physics, fall 2011
Schramm-Loewner evolution (Introduction to mathematical physics), fall 2011
Lecturer
Scope
7 cu.
Type
Advanced studies
Prerequisites
Probability theory (or familiarity with probability and some measure theory) and some complex analysis (including at least conformal maps and Riemann mapping theorem). The material on Function theory III taught in the fall 2010 semester is useful but not required.
Description
The theory of Schramm-Loewner evolutions (SLE) is a topic which combines fruitfully complex analysis with Brownian motion. SLEs are random planar curves which are characterized by certain conformal invariance property.
SLE was introduced by Oded Schramm in 1999 and since then it has been really active field in mathematics. The original motivation of SLE was to study in what way critical statistical physics models are invariant under conformal mappings. Because of conformal invariance, SLEs are the only possible continuum limits of interfaces in two-dimensional statistical physics. The rigorous connection between those random interfaces and SLE is the biggest success of the theory.
This course is aimed for anybody interested in complex analysis, stochastics and/or statistical physics.
On this course we will first spend a couple of weeks giving the necessary background in stochastic calculus (Brownian motion, Ito integral, Ito's formula, stochastic differential equations etc.) which is of course extremely useful tool for many things, also outside this course. Then we recall some background in complex analysis, most likely, more quickly without proofs. Most of the course will be spend introducing and studying SLE and will include the following things:
- Loewner equation
- Schramm-Loewner evolution
- Existence of SLE as a random curve
- Some properties of SLE (fractal properties of SLE, symmetries of SLE,…)
During the course we will also comment on the connection between SLE and the statistical physics, but we don't have time for the full treatment of that topic.
Description by pictures
Realizations of SLE for two different parameter values:
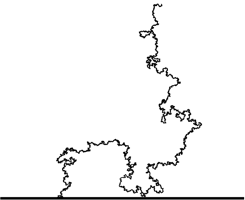
SLE(3)

SLE(6)
Some examples of the models successfully connected to SLE:
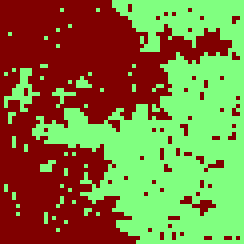
Critical Ising model
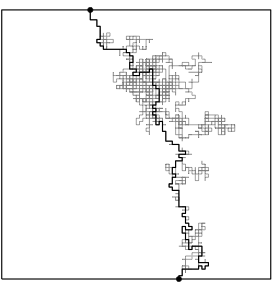
Loop-erased random walk
Lectures
Weeks 36-42 and 44-50, Thursday 14-16 in room C123.
Exams
20.12.2011 Tuesday 12-16
26.1.2012 Thursday 16-20
Bibliography
We will follow mostly
- G. Lawler, Conformally Invariant Processes in the Plane, AMS (2005).
Here are some introductory texts to SLE:
- W. Werner, Random planar curves and Schramm-Loewner evolutions
- S. Smirnov, Towards conformal invariance of 2D lattice models
- G. Lawler, Schramm-Loewner Evolution
Lecture summaries
Short description of each lecture will appear here together with the lecture notes:
- 8.9.2011: Introduction, conditional expected value, definition of Brownian motion. ,
- 15.9.2011: Quadratic variation of Brownian motion, Ito integral.
- 22.9.2011: Martingales, Ito integral as a process.
- 29.9.2011: Ito's formula, Additional topics in stochastic analysis.
- 6.10.2011: Conformal invariance of planar Brownian motion, on conformal mappings.
- 13.10.2011: Basics of conformal maps in the upper half-plane. ,
- 20.10.2011: Loewner equation holds for simple curves.
- 27.10.2011: No lecture on Week 43
- 3.11.2011: Loewner chains, the definition of Schramm-Loewner evolution, Schramm's principle.
- 10.11.2011: Phases of SLE. (only minor changes),
- 17.11.2011: More example calculations with Schramm-Loewner evolution.
- 24.11.2011: SLE(kappa,rho), locality of SLE(6), continuity of the chordal SLE.
- 1.12.2011: Continuity of the chordal SLE.
- 8.12.2011: Continuity of the chordal SLE, dimension of SLE. (Final version of the lecture notes. Updated: noon Dec 15.)
- 15.12.2011: Last lecture.
In January or February 2012, there will be a seminar talk on the subject "How to show that a discrete random curve converges to SLE?".
Exercises
- 13.9.2011:
- 20.9.2011:
- 27.9.2011:
- 4.10.2011:
- 11.10.2011:
- 18.10.2011:
- 1.11.2011:
- 8.11.2011:
- 15.11.2011:
- 22.11.2011:
- 29.11.2011:
- 13.12.2011:
Registration
Did you forget to register? What to do.
Exercise groups
Group | Day | Time | Place | Instructor |
|---|---|---|---|---|
1. | Tue | 16.15 | C123 | Miika Nikula |