Hauptmann, Andreas
Andreas Hauptmann
Tohtorikoulutettava
Doktorand
Doctoral student
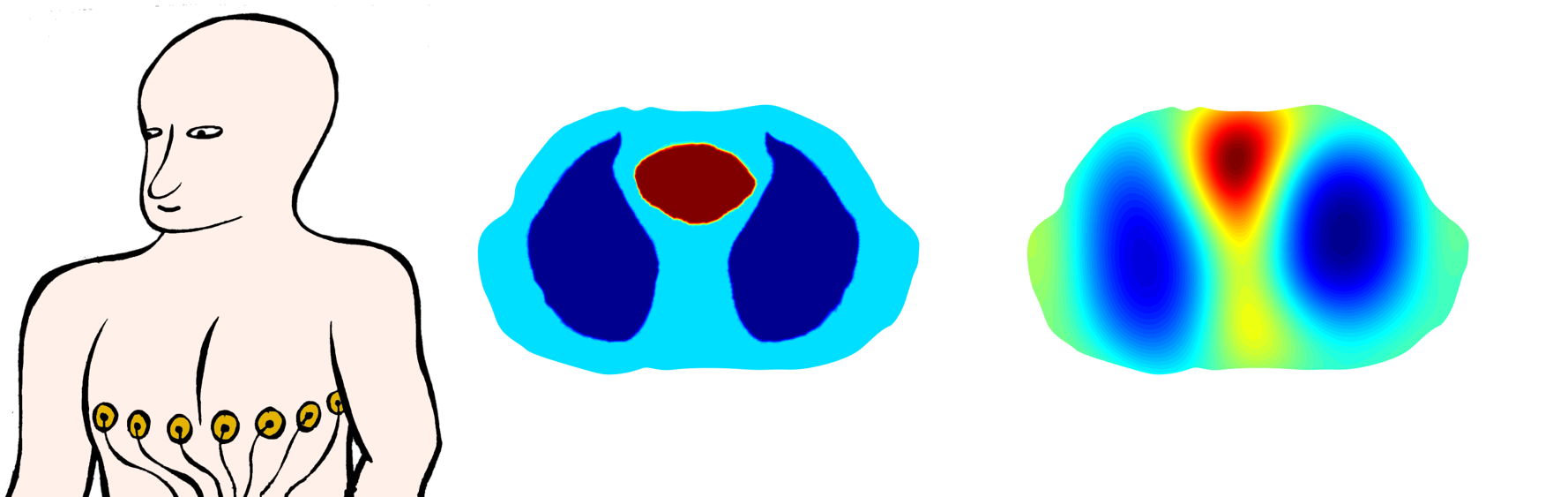

I have finished my PhD and moved to a new position at the Centre for Medical Image Computing in University College London. (Link)
As an applied mathematician with a focus on computational mathematics I am interested in inverse problems with real measurement data, in particular applications to medical imaging.
In my PhD thesis I investigate the problem of partial-boundary data in electrical impedance tomography (EIT), a non-invasive imaging modality that seeks to recover the conductivity of a body from electrical measurements taken at its surface. EIT is a highly non-linear ill-posed inverse problem that needs careful analysis of the underlying mathematics.My work in EIT focuses on developing noise robust sharp images from limited boundary data (e.g. a patient lying on their back or faulty electrodes).
Furthermore, I am interested in dynamic imaging and regularization strategies. That is given a set of measurements at several time instances, we seek to compute a reconstruction that uses all time dependent information. These problems arise for instance in X-ray computed tomography of a moving object.
Opetus / Undervisning / Teaching
Lecturer: Computational methods for Integral Equations, Spring 2017
Teaching Assistant: Bayesian inversion, Spring 2016
Teaching Assistant: Inverse Problems, Spring 2015
Publications
(submitted) M. Burger, H. Dirks, L. Frerking, A. Hauptmann, T. Helin, S. Siltanen, A Variational Reconstruction Method for Undersampled Dynamic X-ray Tomography based on Physical Motion Models.
(accepted) A. Hauptmann, Approximation of full-boundary data from partial-boundary electrode measurements. To appear in Inverse Problems.
2017 M. Alsaker, S. Hamilton, and A. Hauptmann, A Direct D-bar Method for Partial Boundary Data Electrical Impedance Tomography with A Priori Information. Inverse Problems and Imaging, 11(3), pp. 427 - 454.
2017 A. Hauptmann, S. Santacesaria, S. Siltanen, Direct inversion from partial-boundary data in electrical impedance tomography. Inverse Problems 33(2), 025009.
2014 S. Hamilton, A. Hauptmann, and S. Siltanen, A Data-Driven Edge-Preserving D-bar Method for Electrical Impedance Tomography. Inverse Problems and Imaging, 8(4), pp. 1053-1072.
2014 K. Hämäläinen, L. Harhanen, A. Hauptmann, A. Kallonen, E. Niemi, and S. Siltanen, Total variation regularization for large-scale X-ray tomography. International Journal of Tomography and Simulation 25(1), pp. 1-25.
Yhteystiedot
Huone: B421
Osoite: PL 68 (Gustaf Hällströmin katu 2b)
00014 Helsingin yliopisto
Sähköposti: andreas.hauptmann 'at' helsinki.fi
Kontaktinformation
Rum: B421
Adress: PB 68 (Gustaf Hällströms gata 2b)
00014 Helsingfors universitet
Epost: andreas.hauptmann 'at' helsinki.fi
Contact information
Room: B421
Address: P.O. Box 68 (Gustaf Hällströmin katu 2b)
FI-00014 University of Helsinki
Email: andreas.hauptmann 'at' helsinki.fi