Daniel Meyer's Pictures

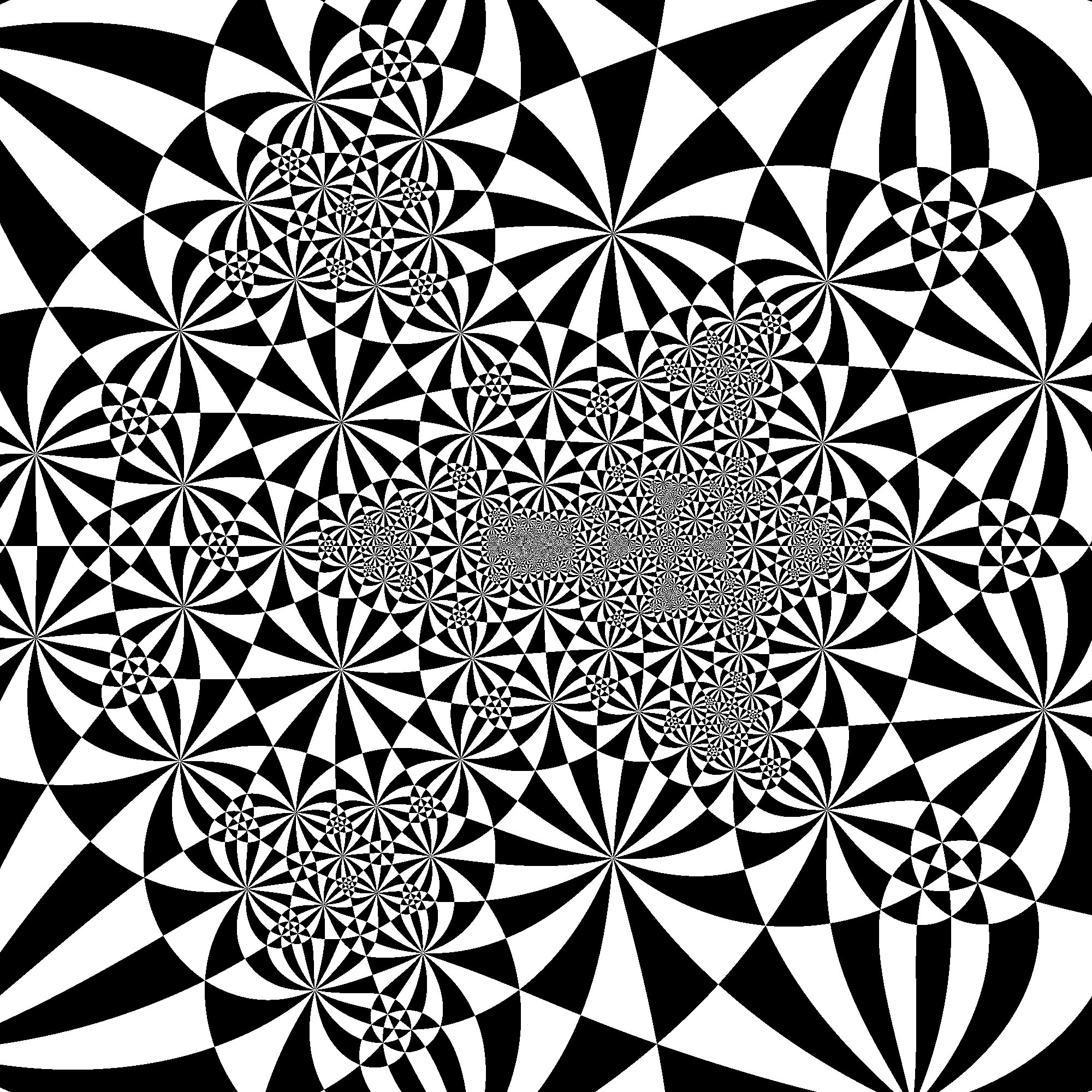
Conformal tilings

Conformal tilings are tilings of the plane or the sphere with the following properties. Each tile is an n-gon where each side is an analytic arc, i.e., the image of a line segment by an analytic map. Furthermore two tiles which share such an analytic arc are conformal reflections along this arc. More precisely if two tiles X,Y share an arc a, then the analytic map f: a → I \subset \R extends to X and Y; f(X), f(Y) are reflections of each other along the real line. In laymans terms: put a curved mirror on one edge, you see the neighboring tile as a reflection. Thus each tile contains the whole information to recover the whole tiling. Conformal tilings were introduced by Bowers Stephenson.

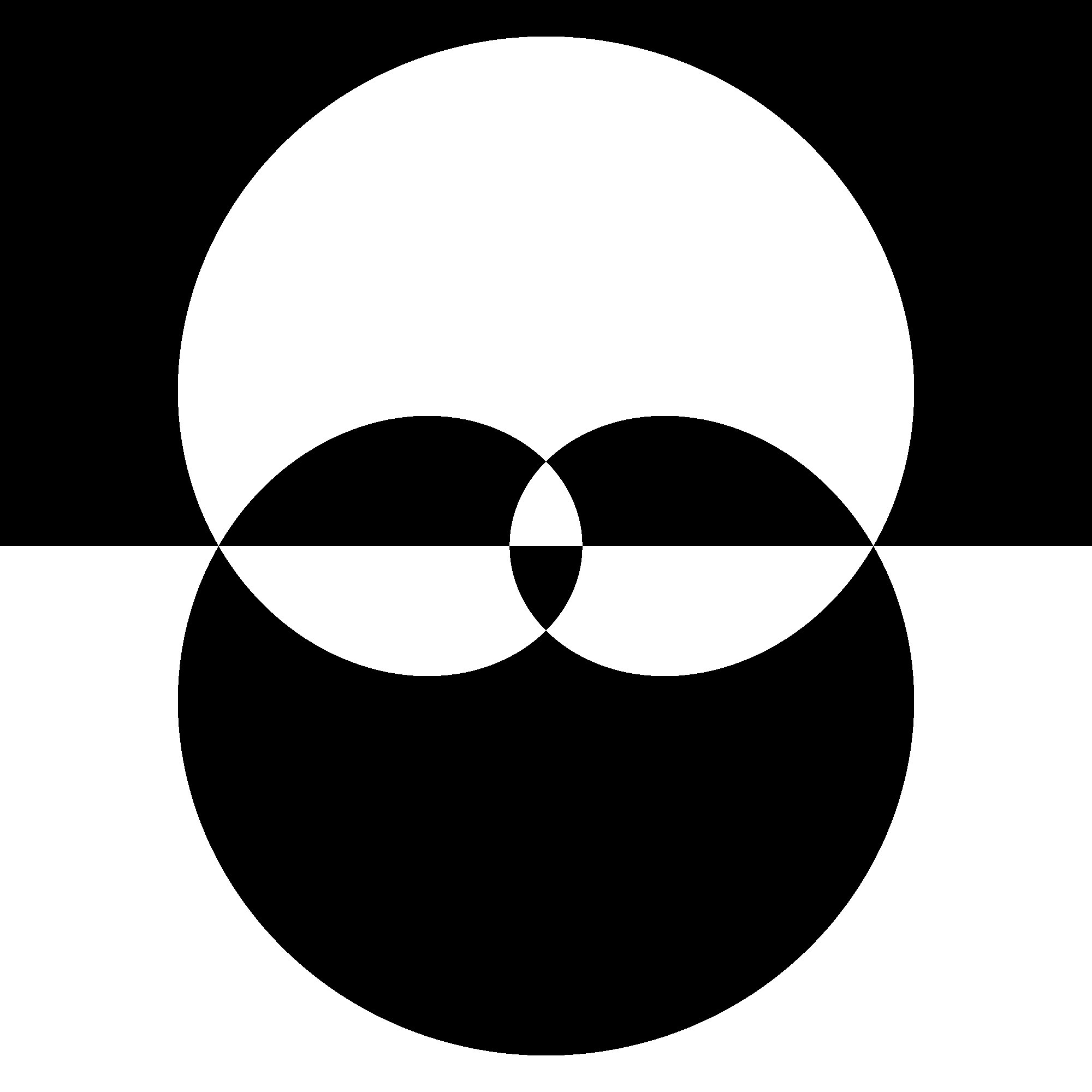
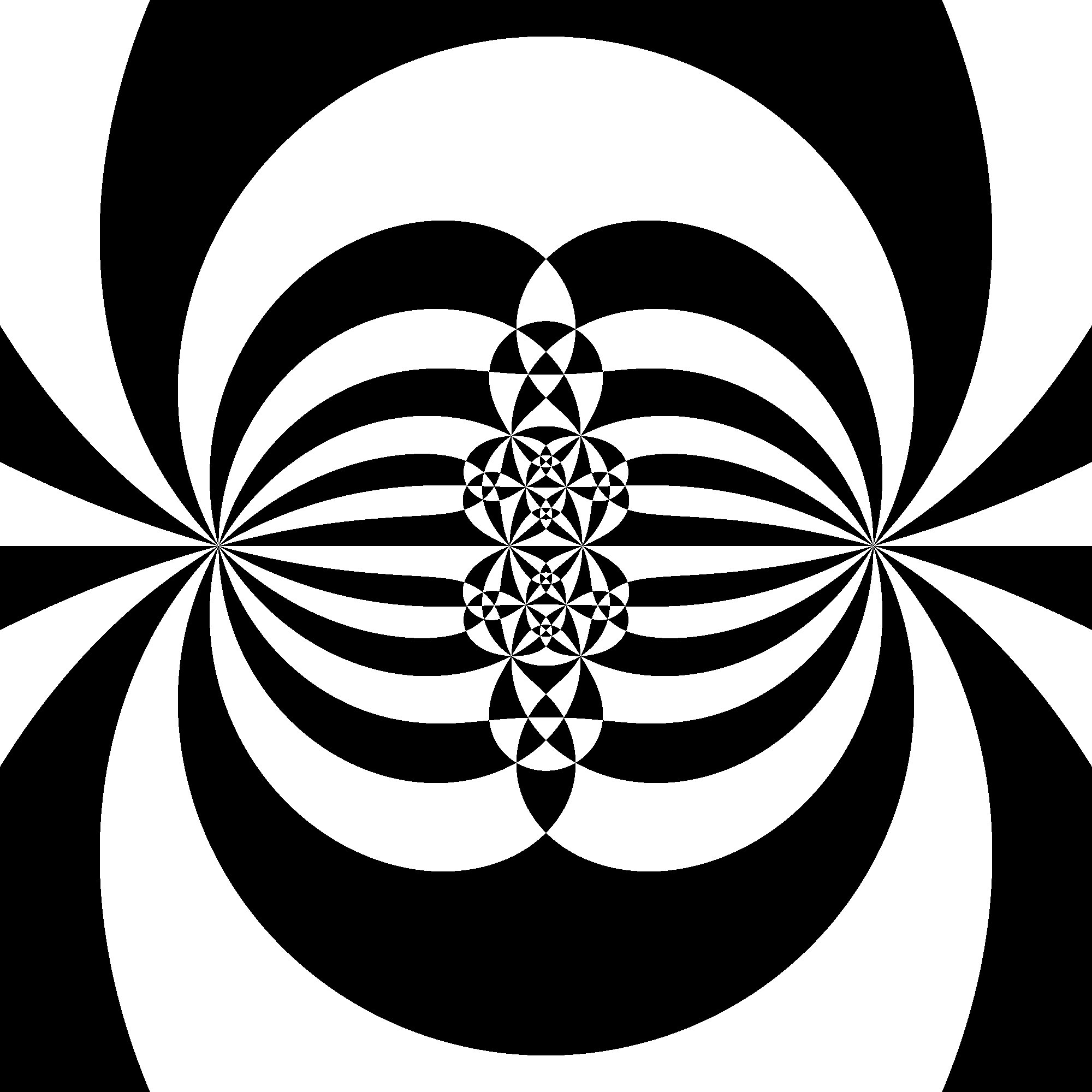
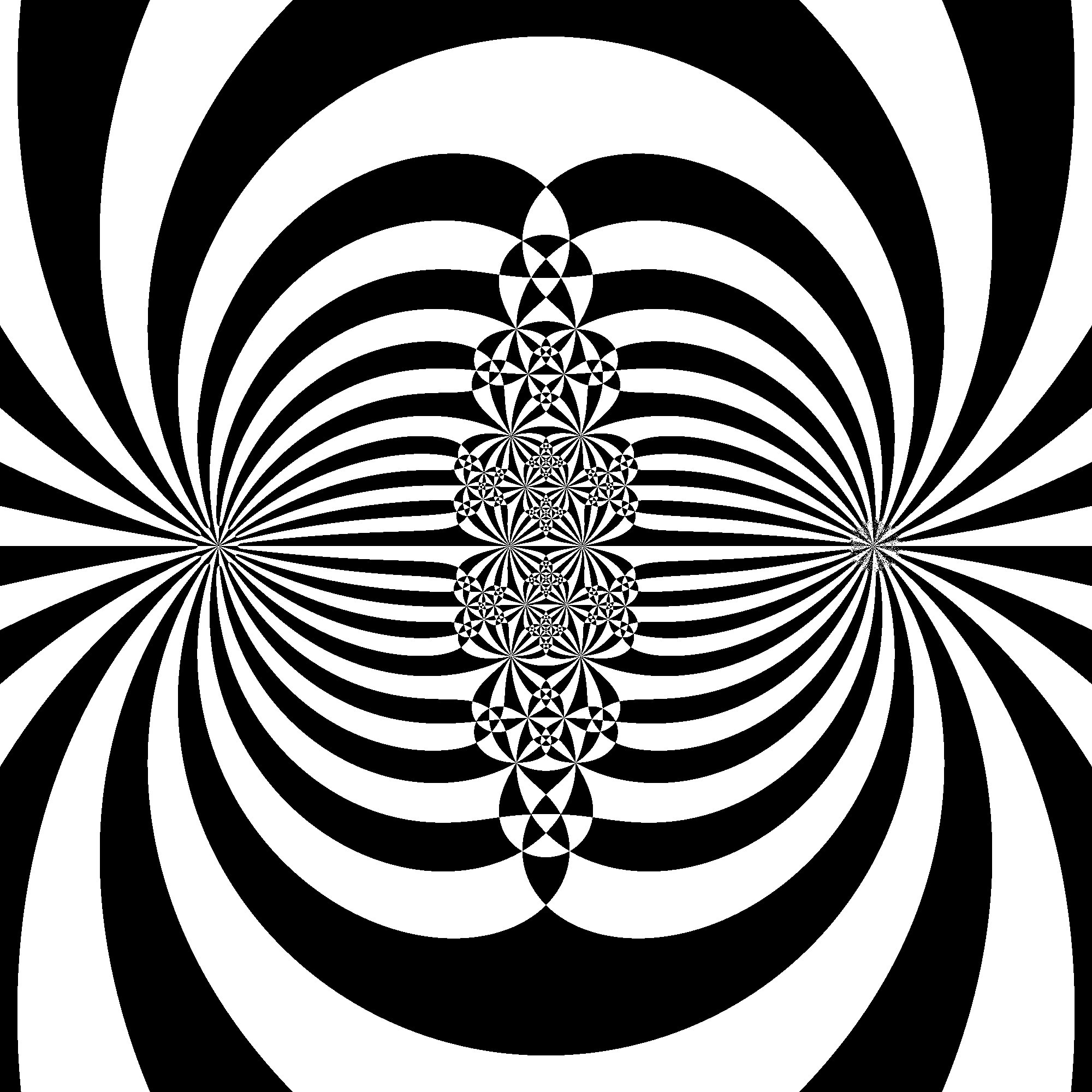
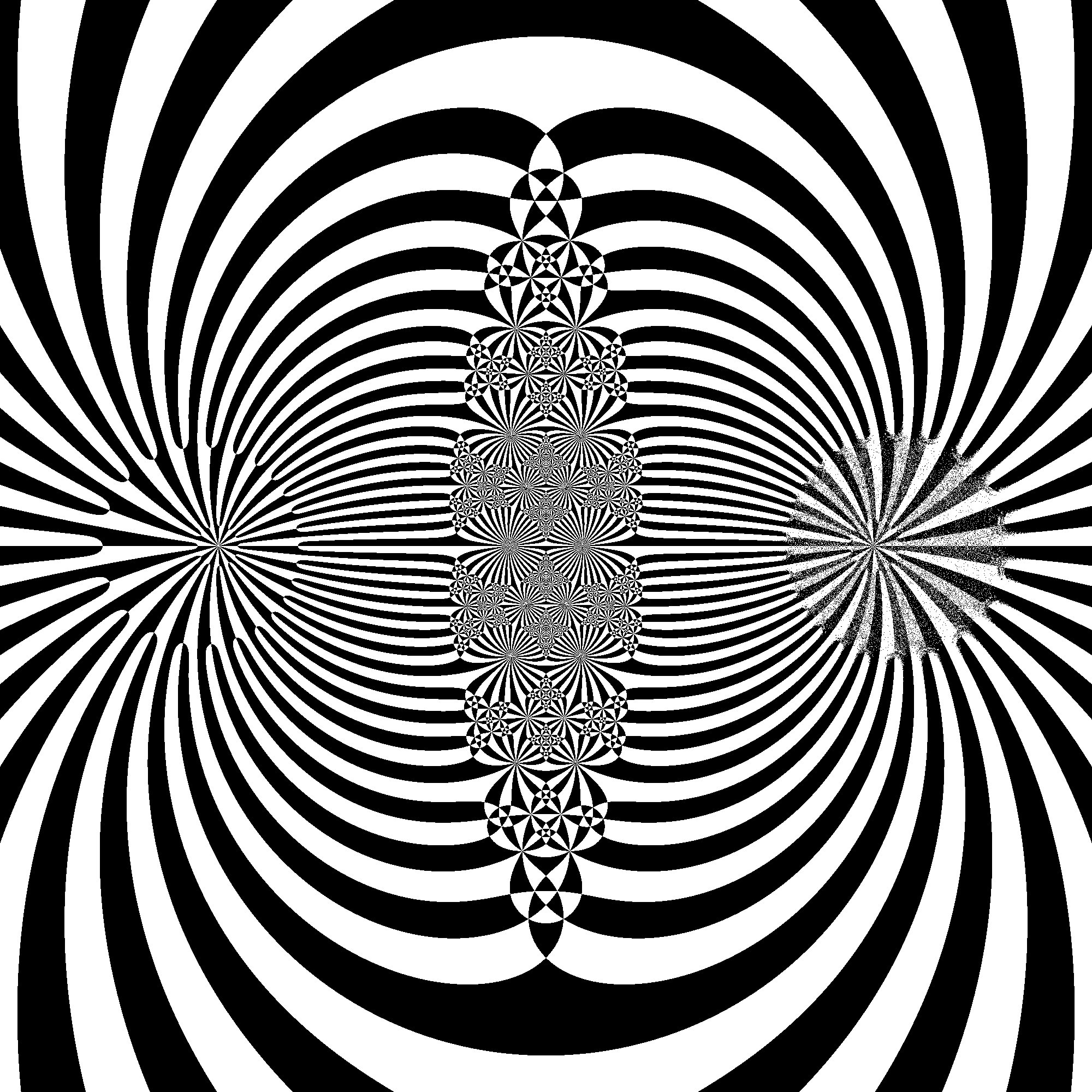
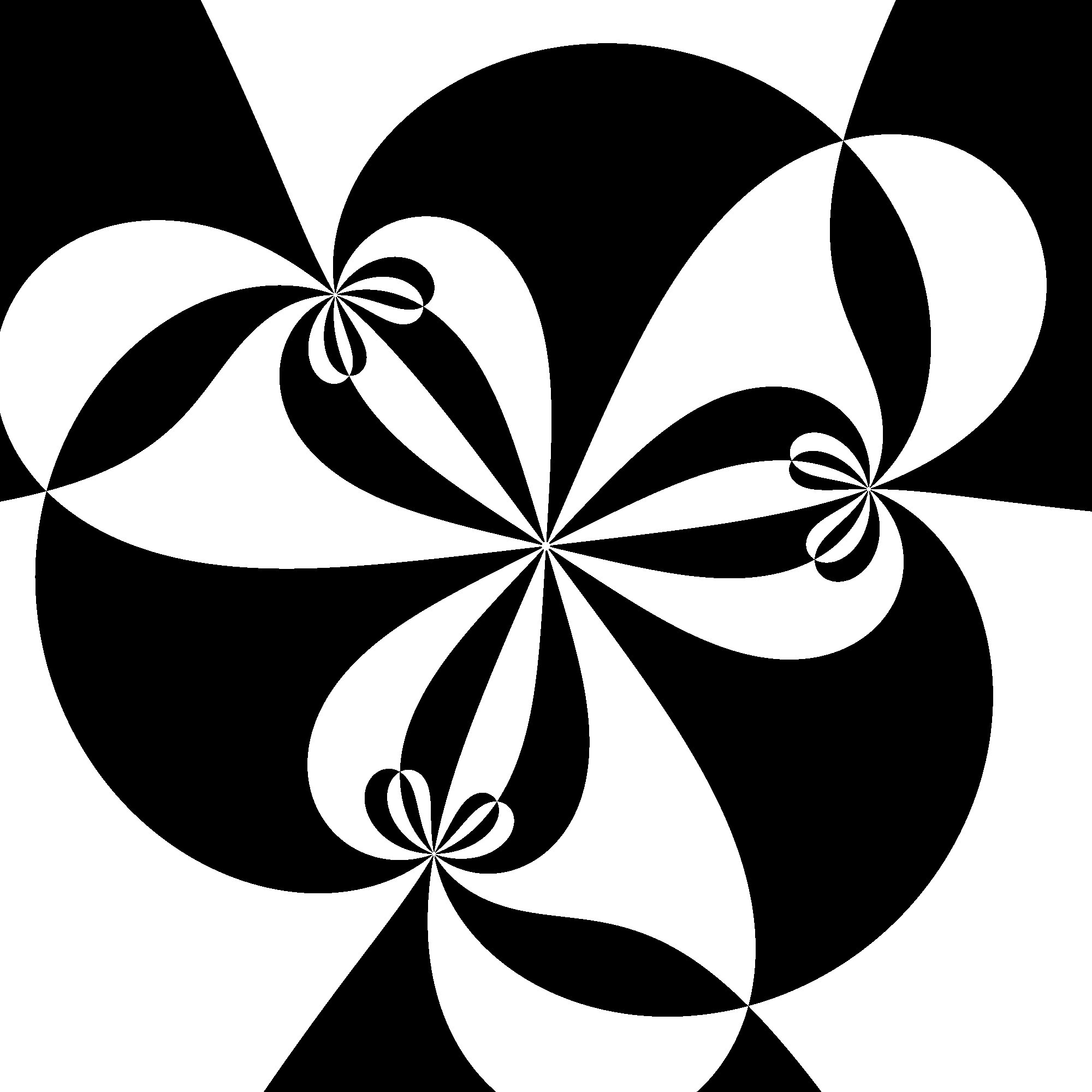
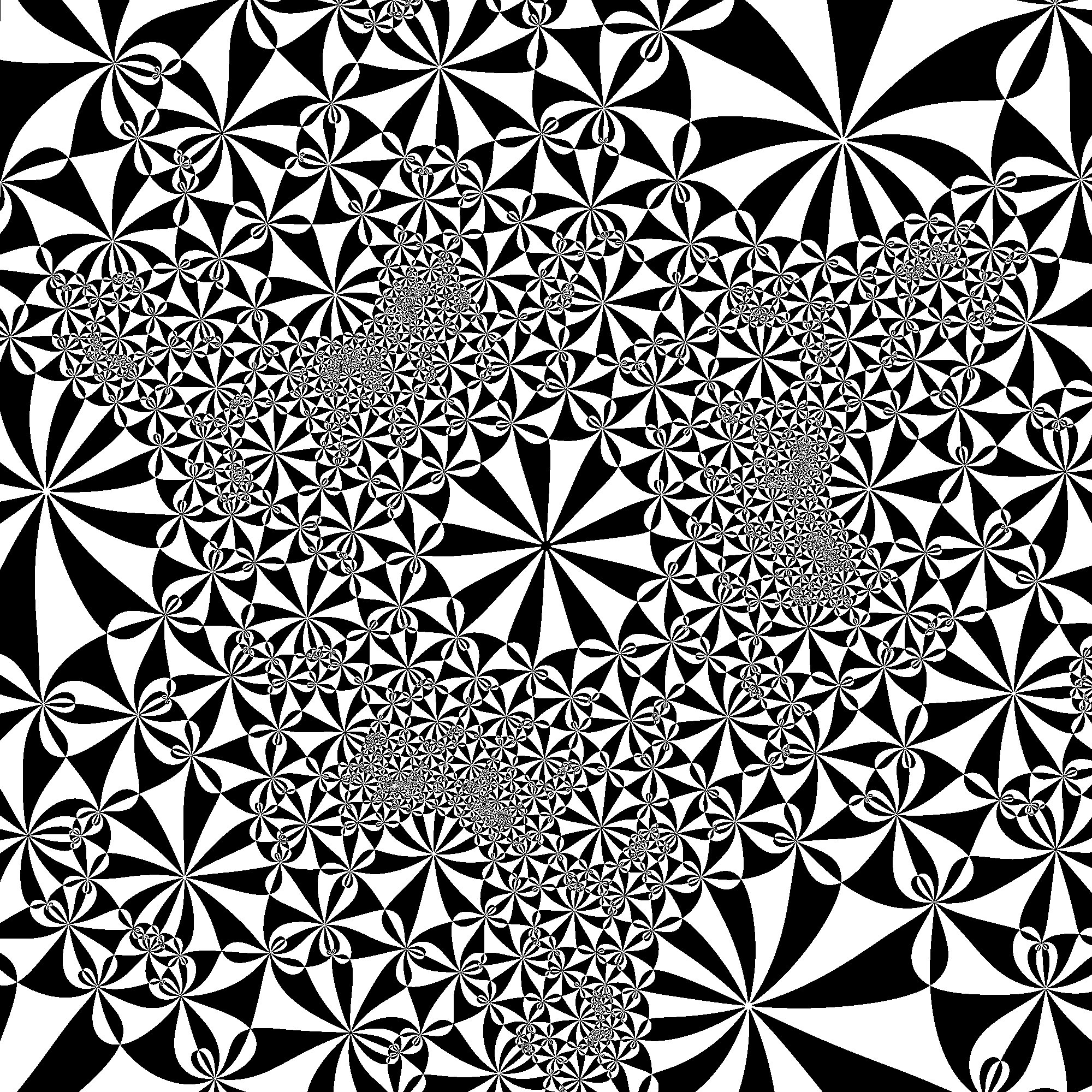
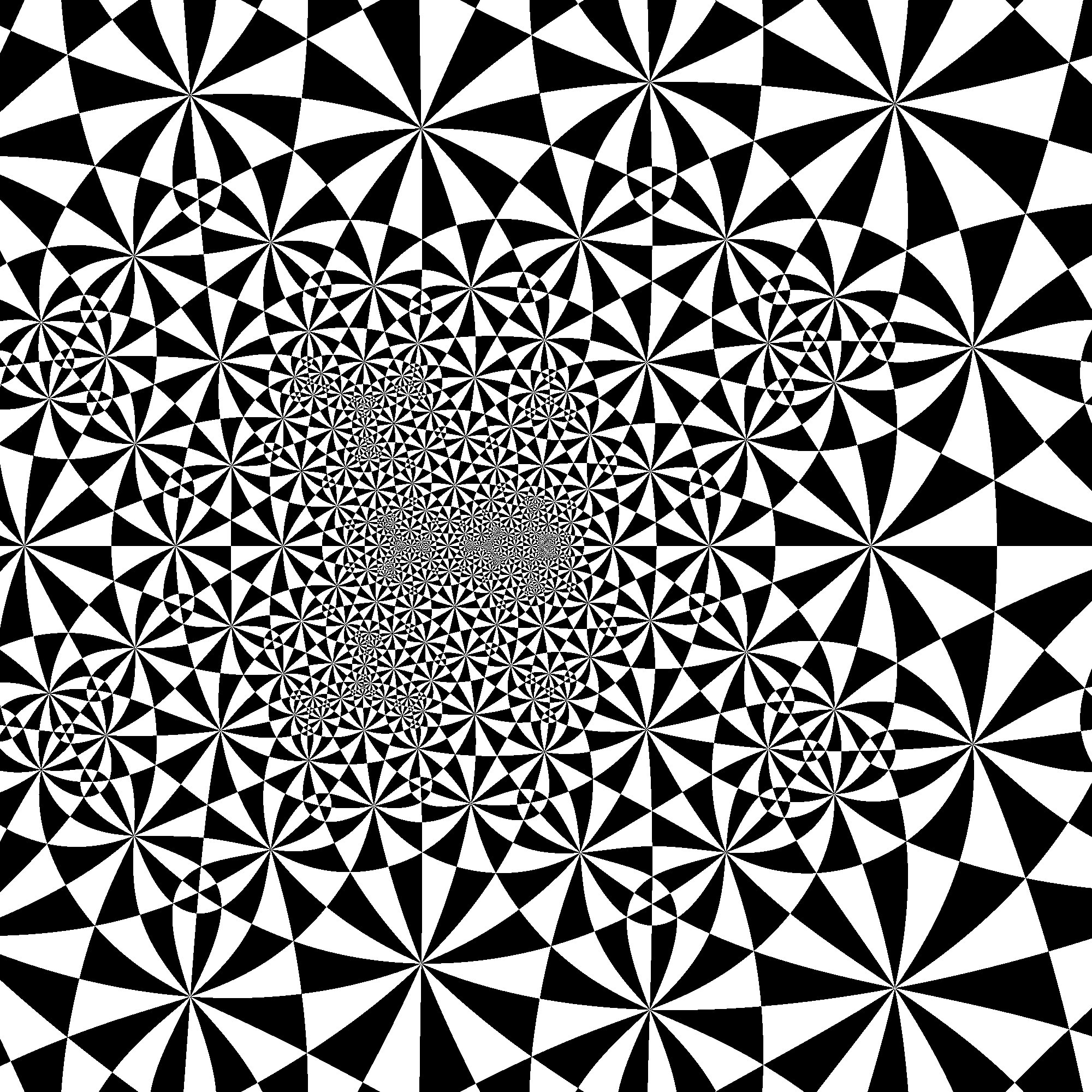
z^2 -1

These conformal tilings are obtained by iteration of z^2-1. The pictures are just preimages of the upper and lower half plane under iteration.

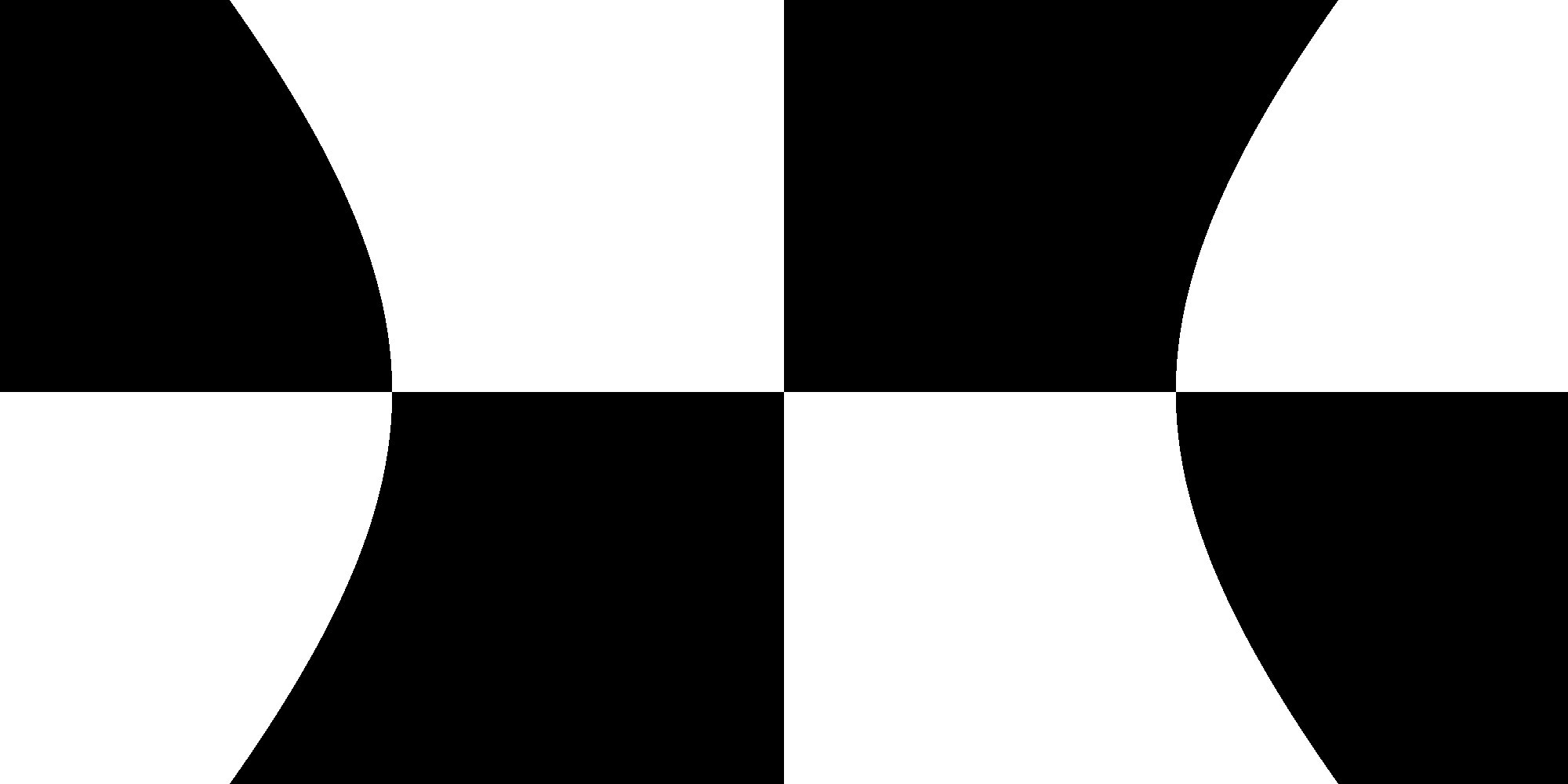
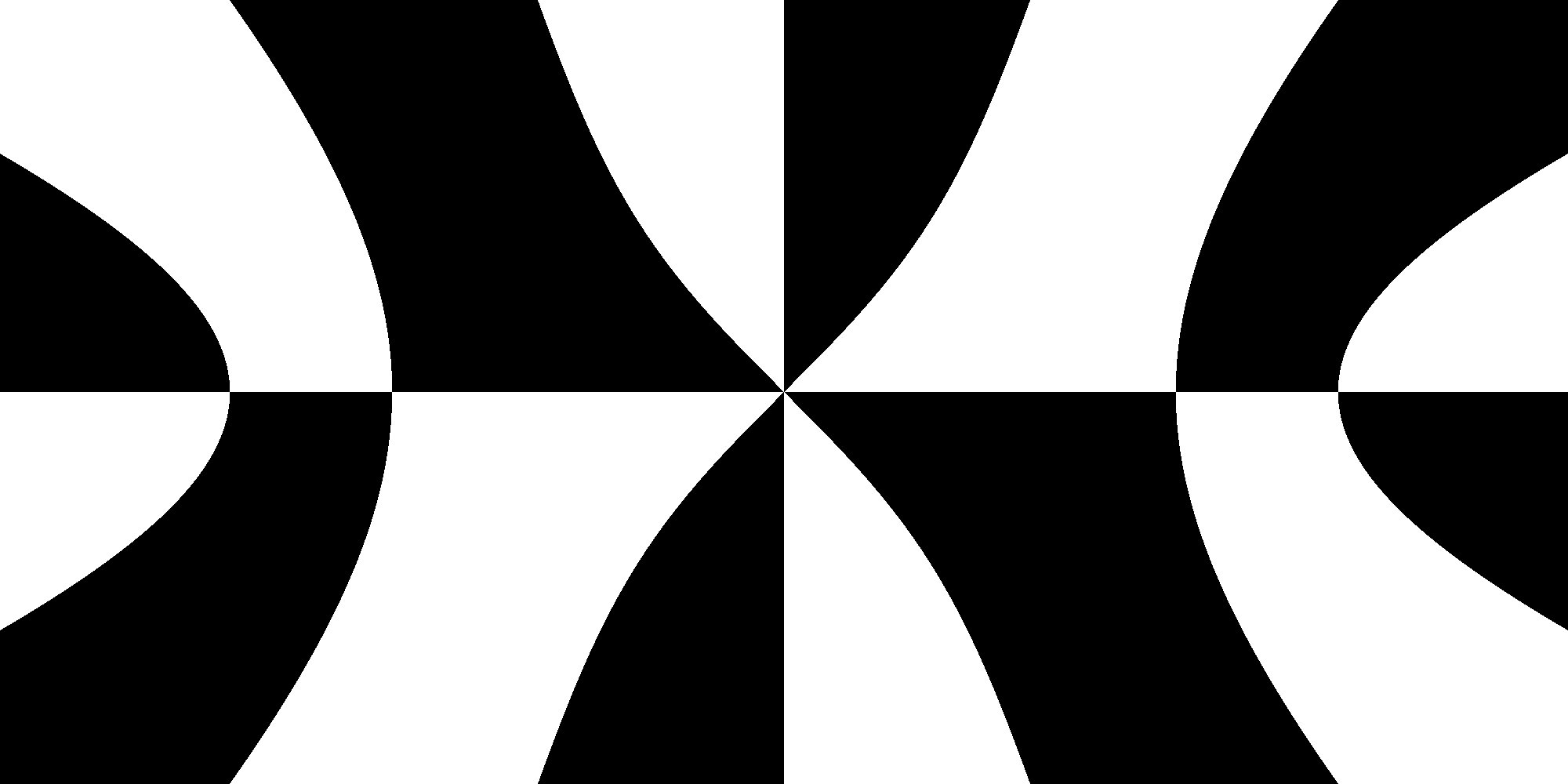
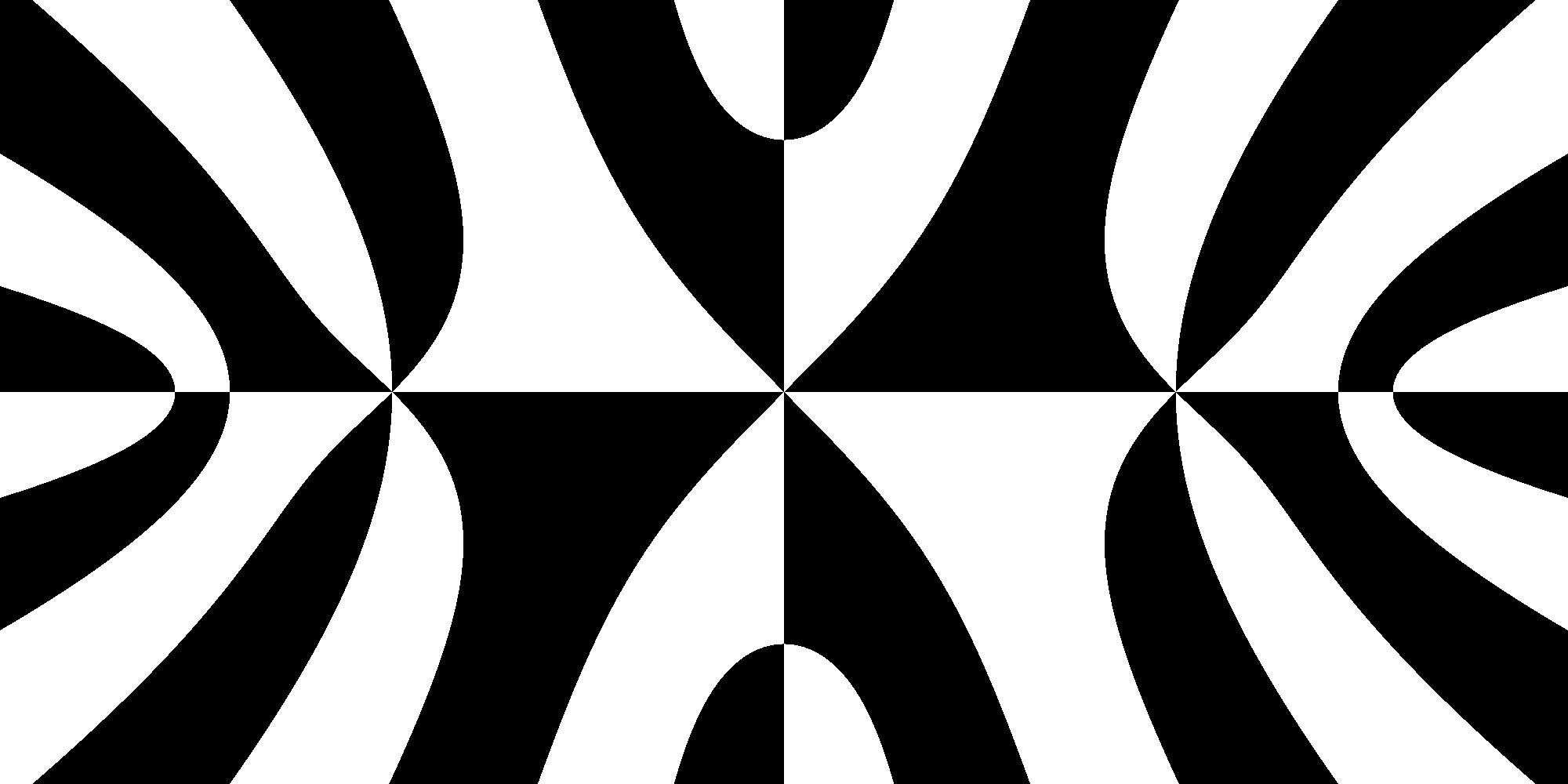
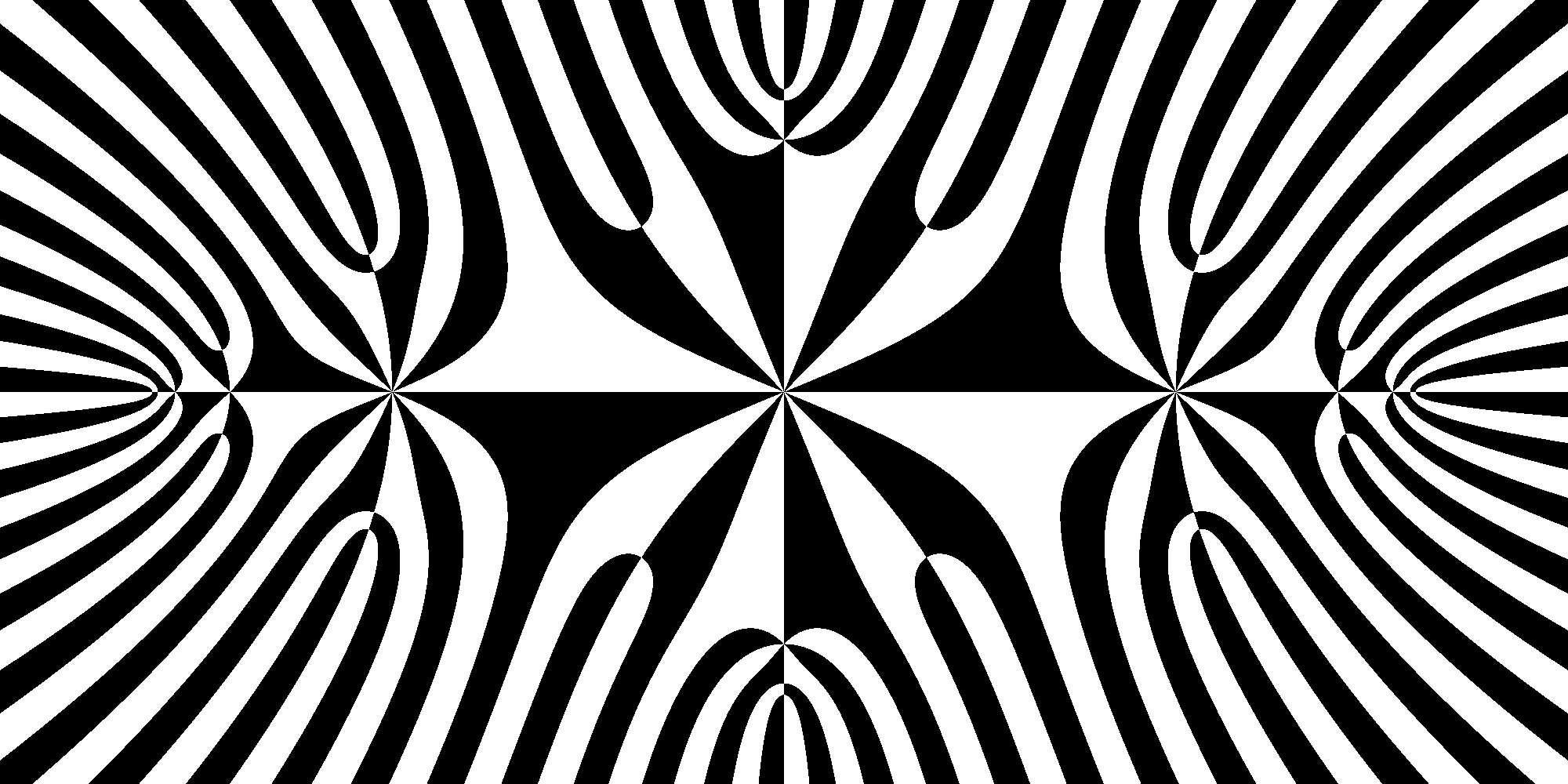
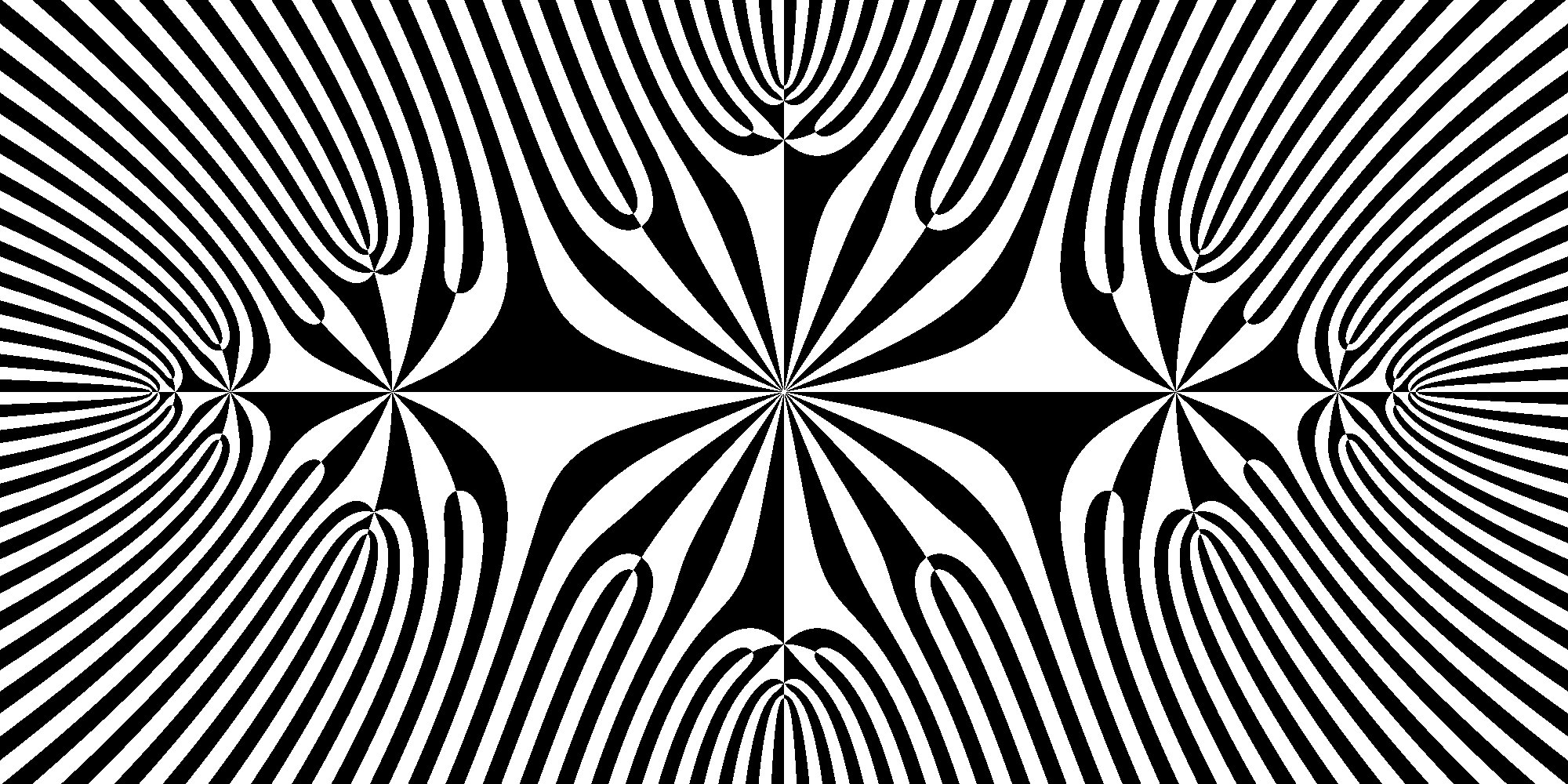
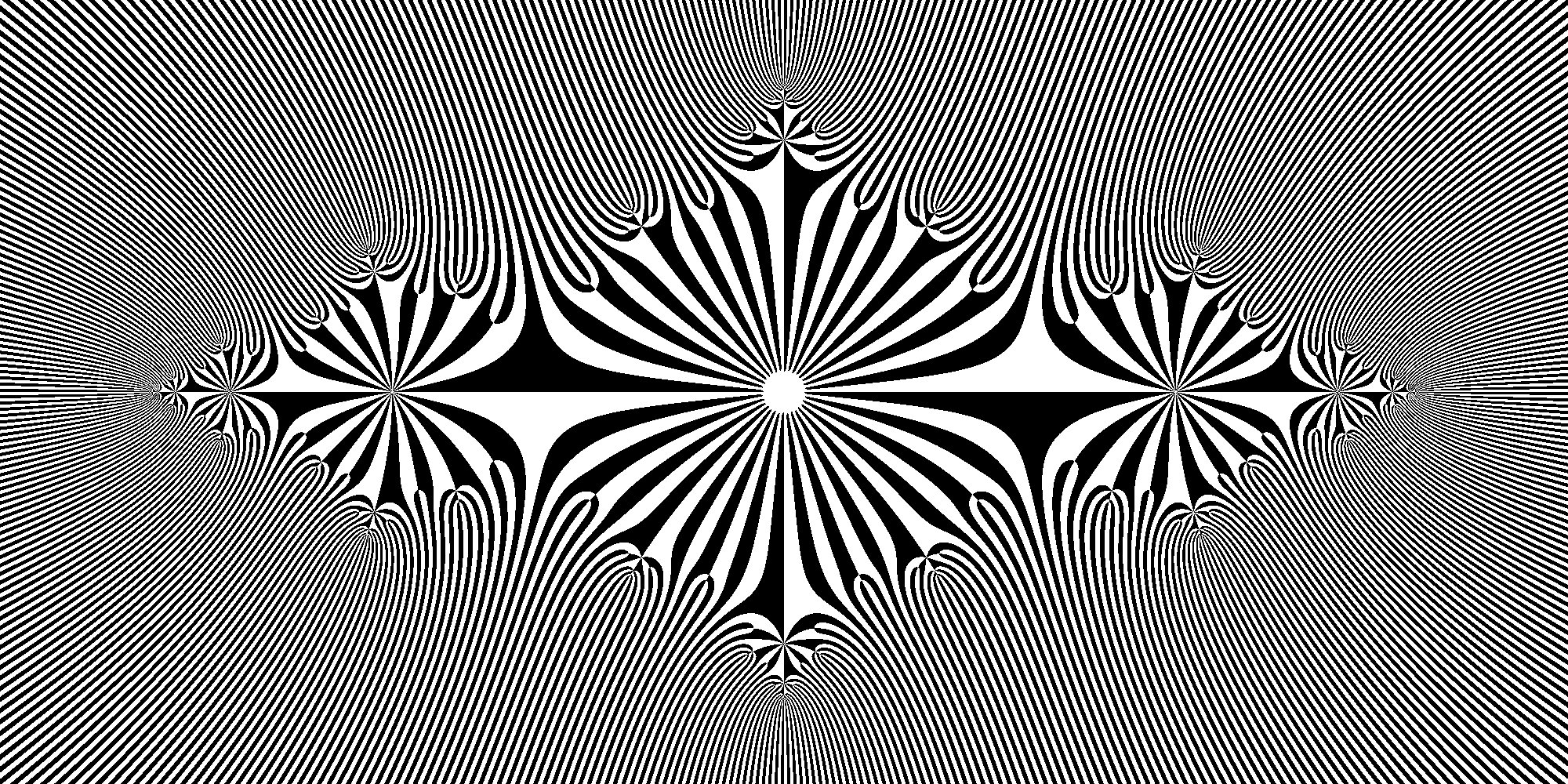
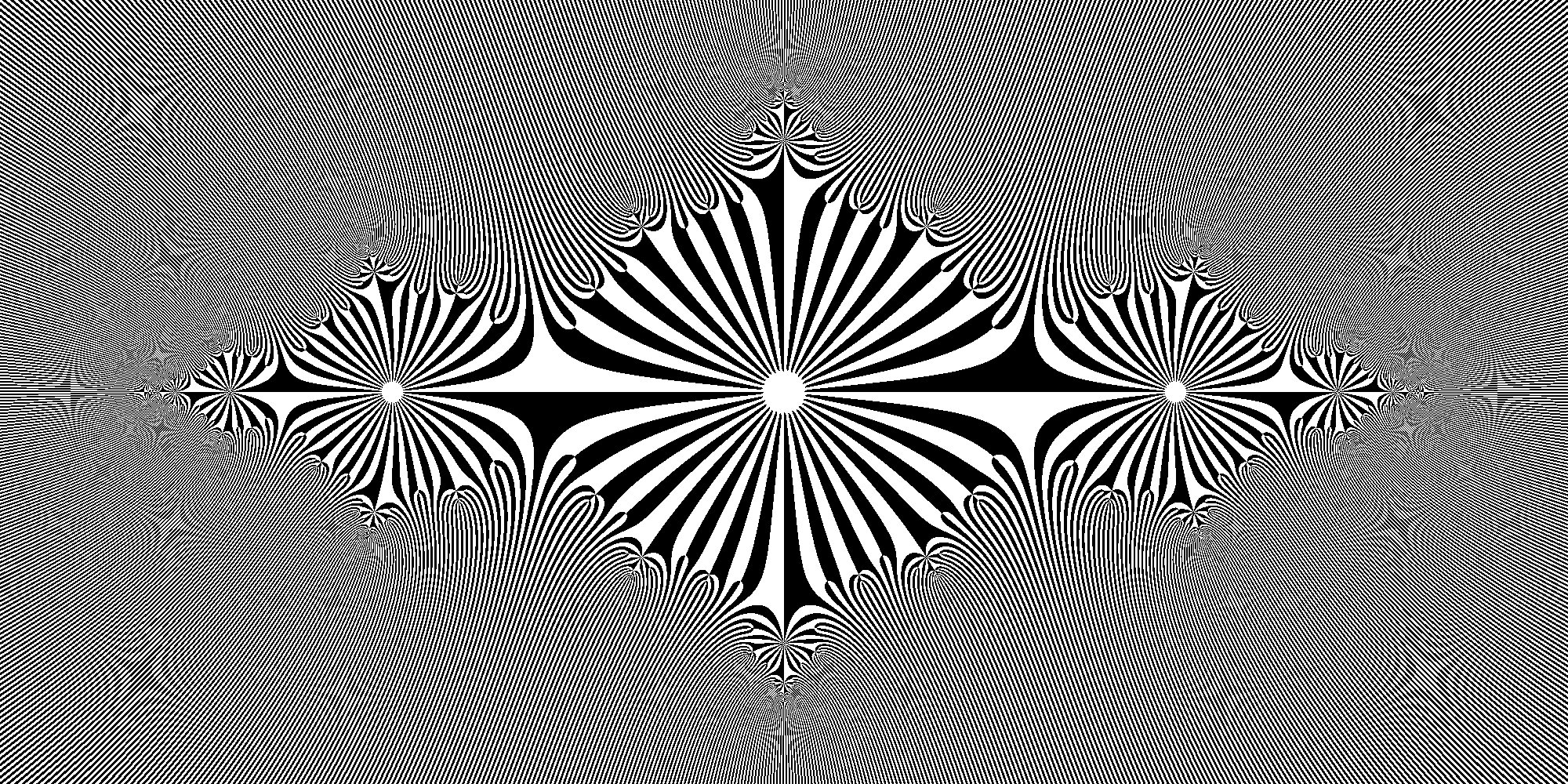
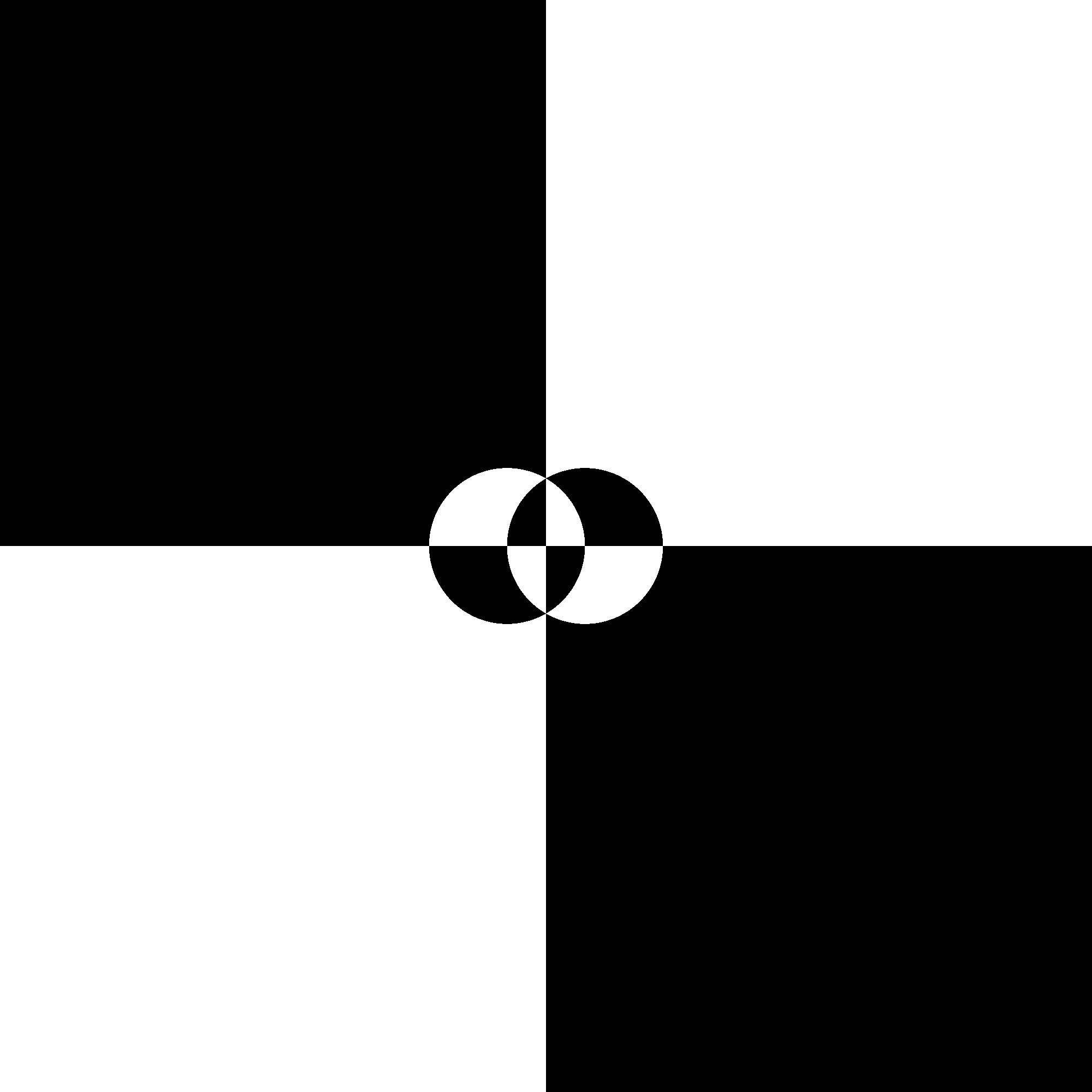
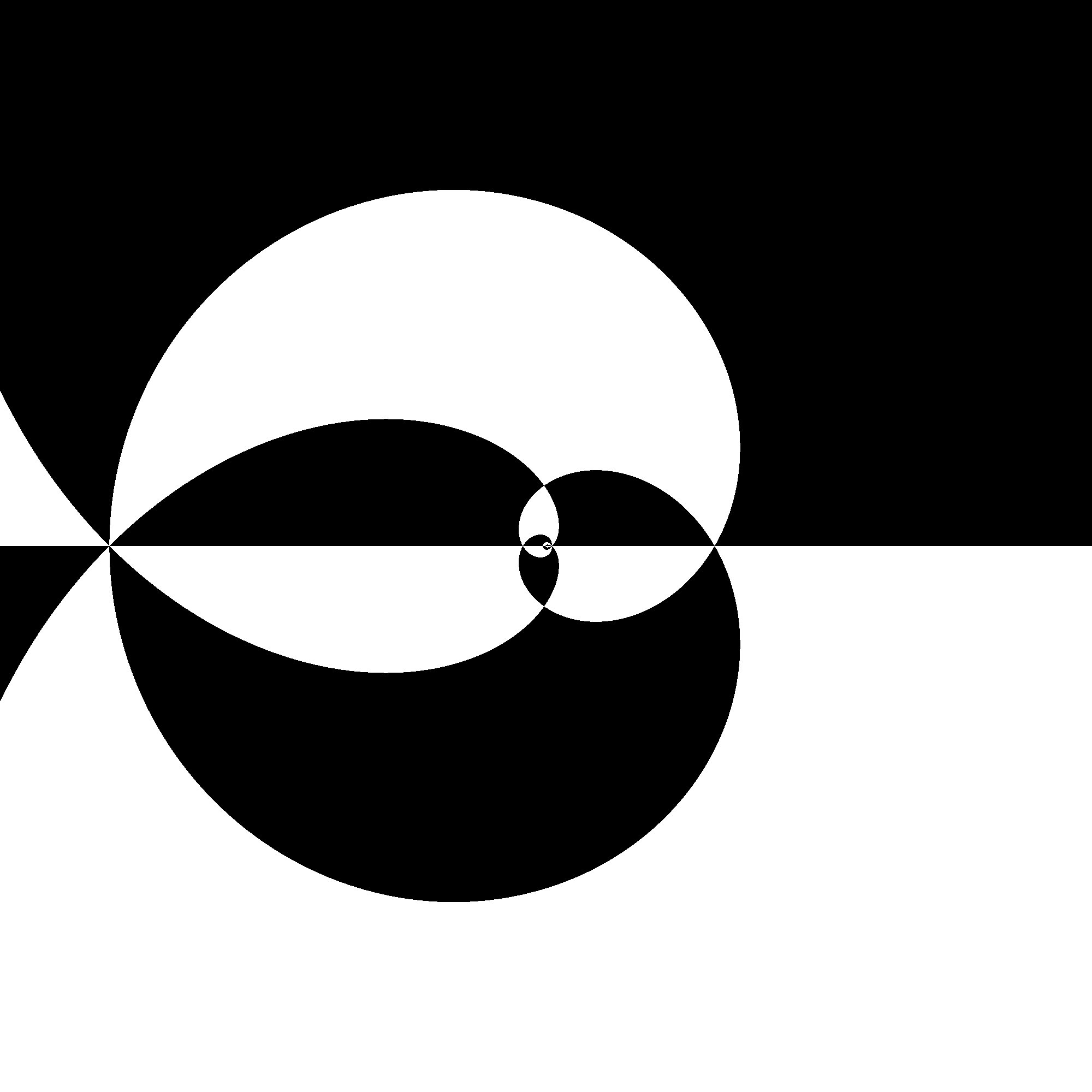
Barycentric subdivision

The barycentric subdivision divides a triangle along the bisectors in 6. This can then be iterated. Here we start with two triangles that are glued along their boundaries to form (topologically) a sphere. The pictures show the obtained conformal tilings. They were obtained using the map r(z)= 1- 54(z^2-1)^2/(z^2+3)^3 that encodes this subdivision, obtained by Cannon-Floyd-Kenyon-Parry.

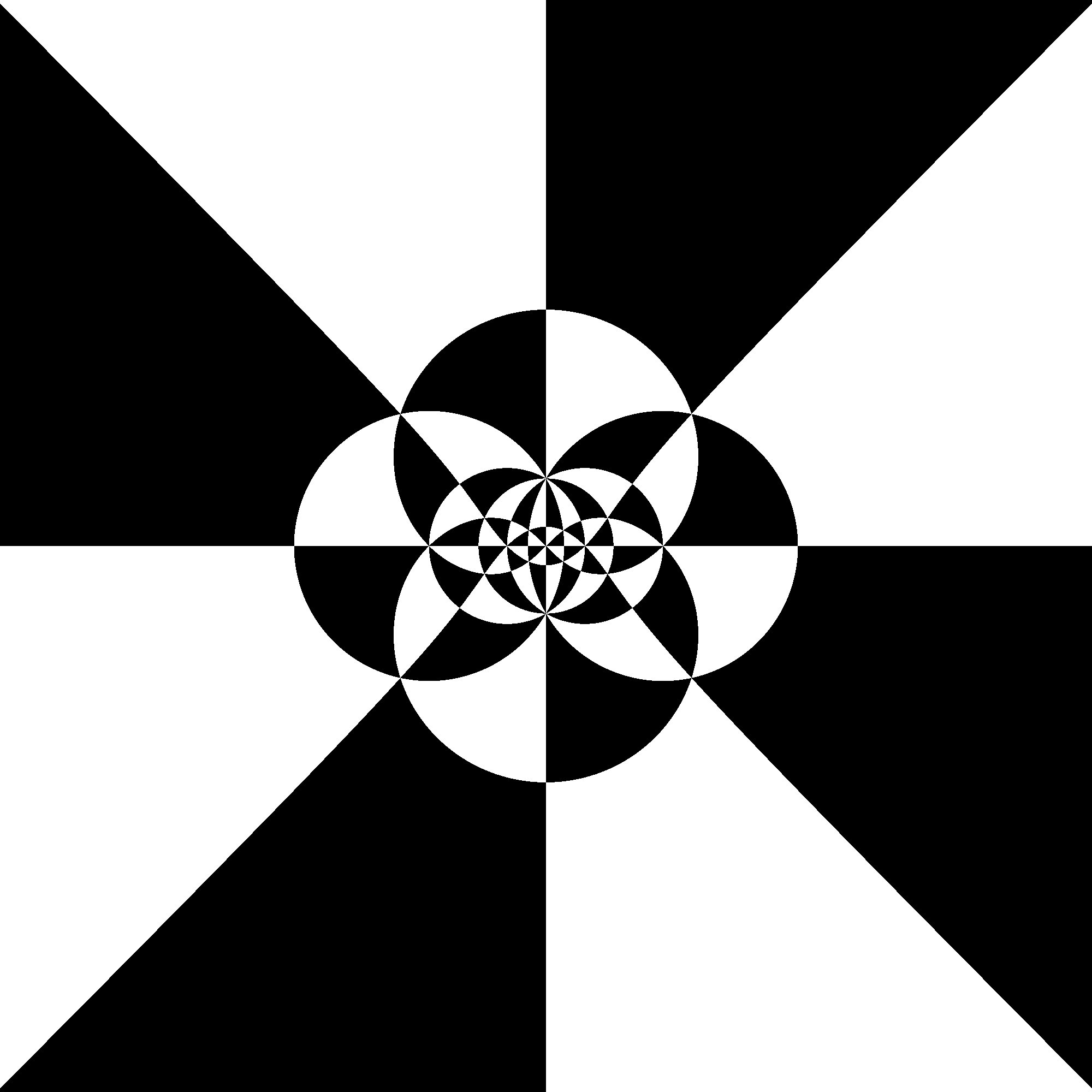
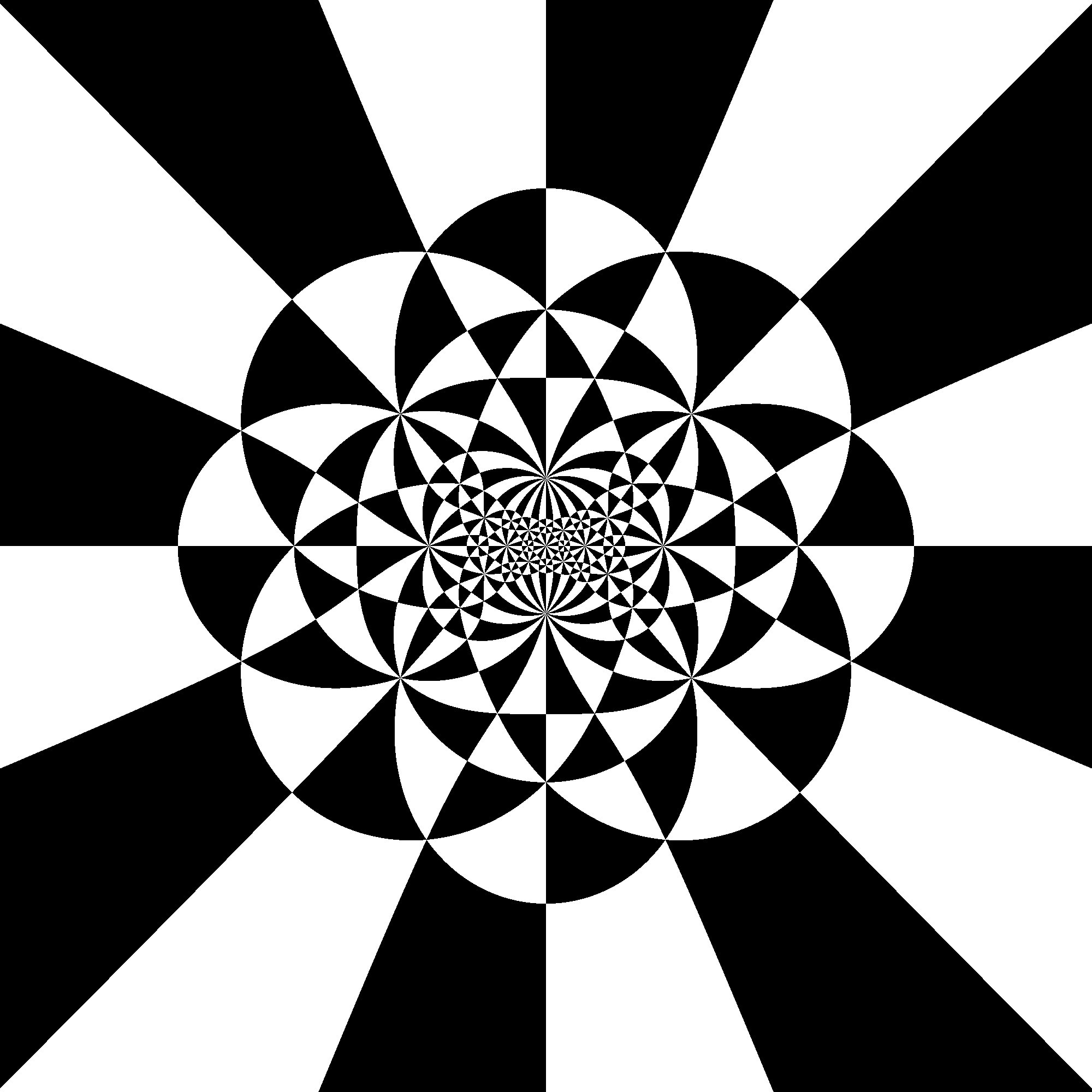
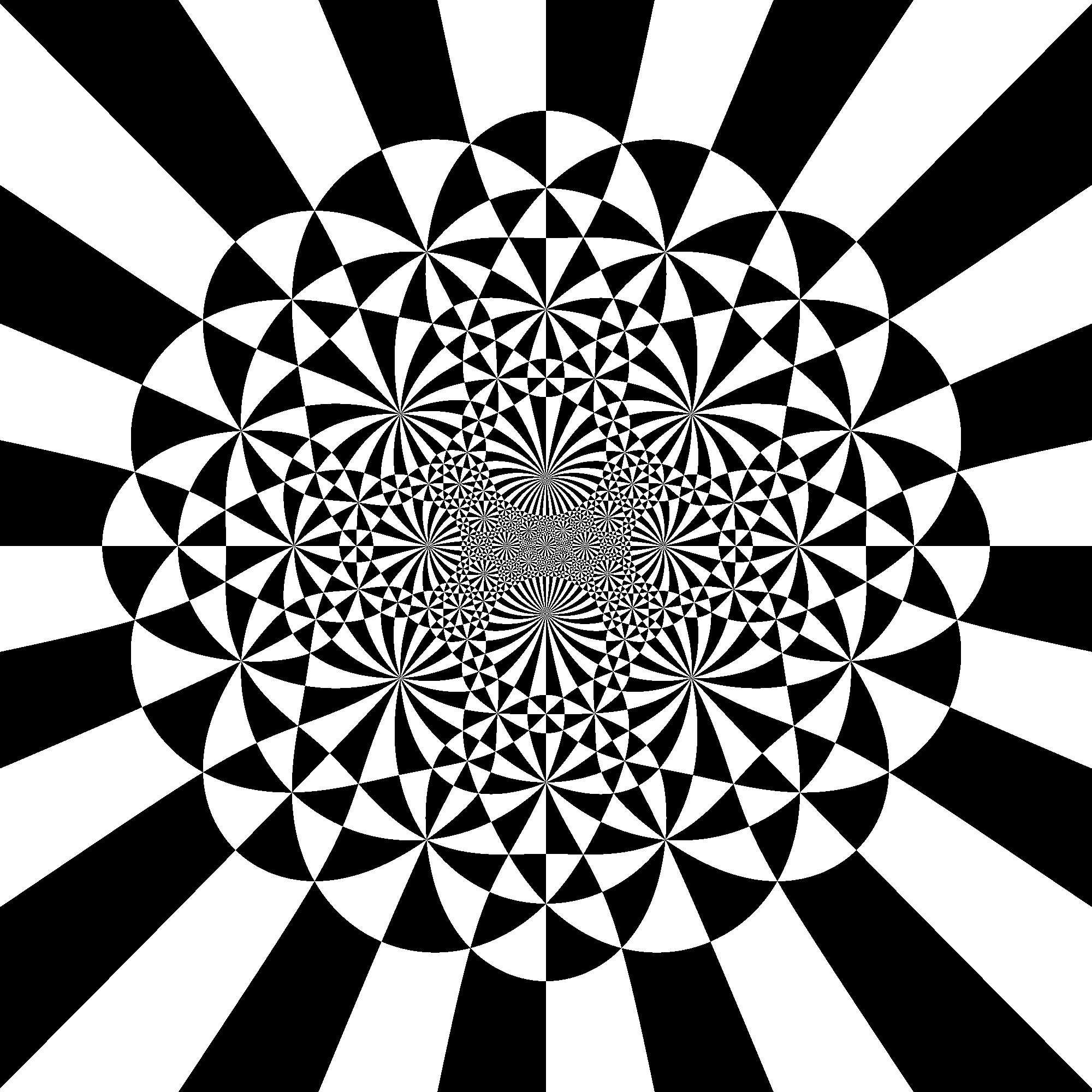
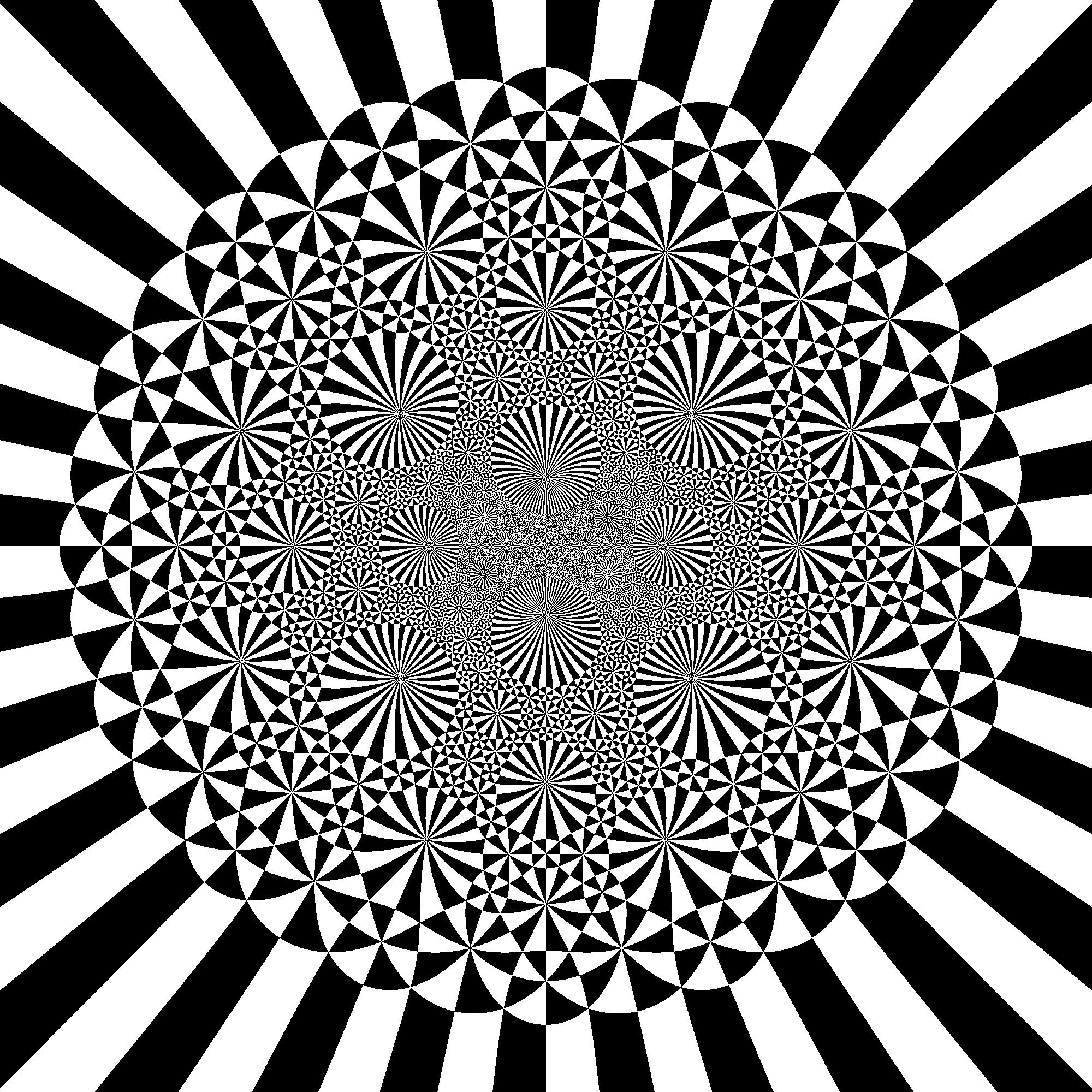
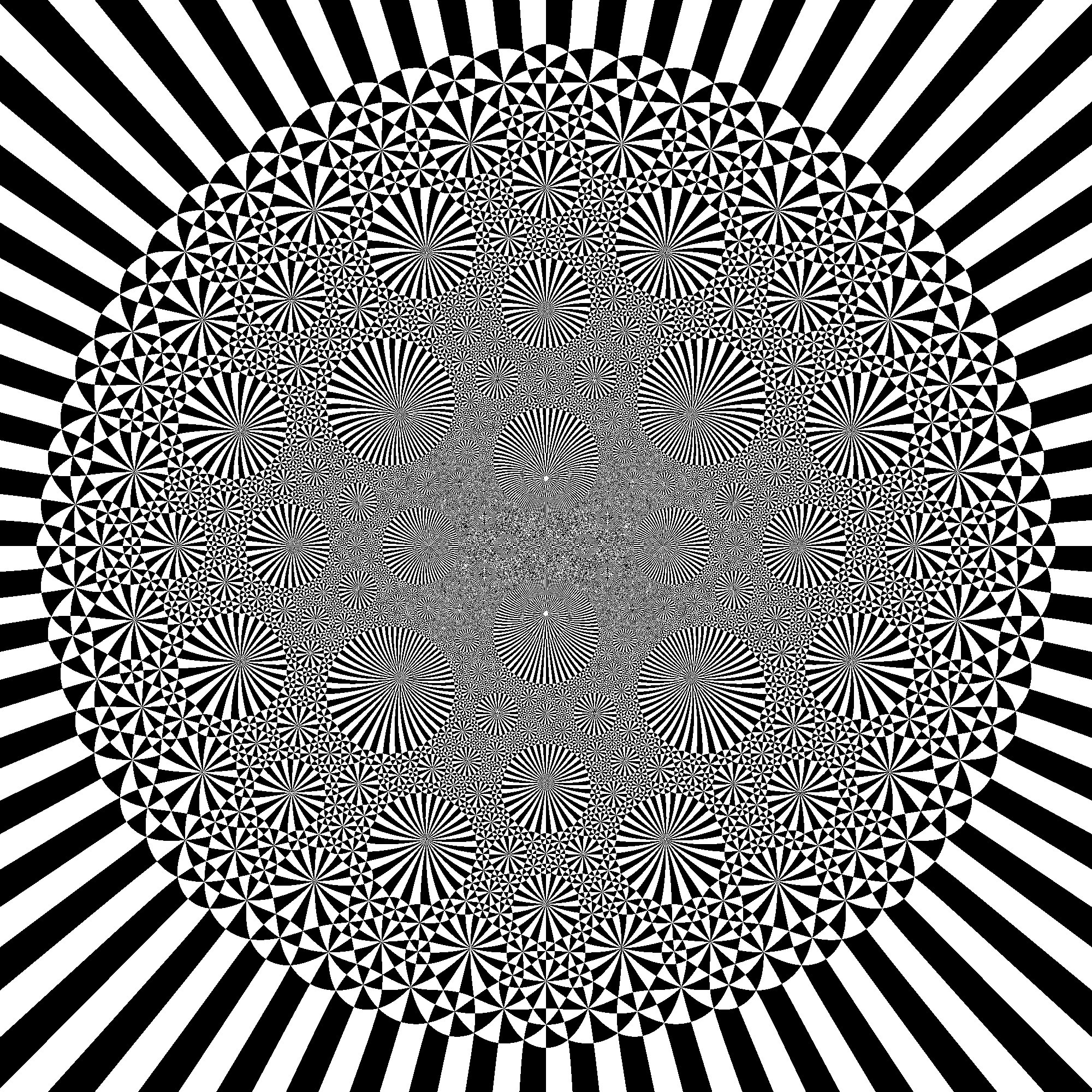
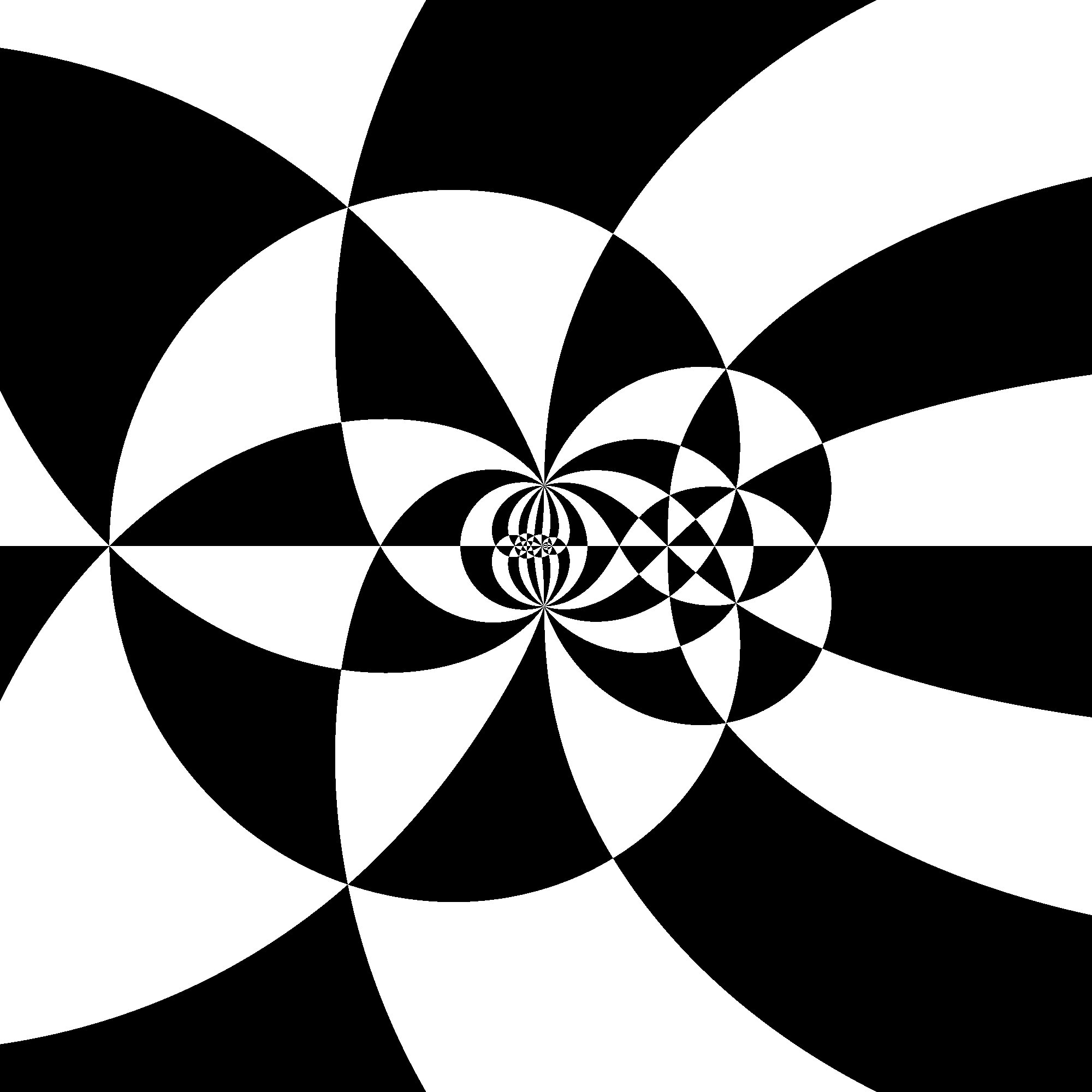
Flap Space

Here is another example obtained as follows. We start with the sphere that is obtained from glueing two triangles together. They are colored black and white. The iteration is as follows. Each black triangle is divided along one bisector in two. Each white triangles is first divided along a bisector in two, in this new edge two triangles (a flap) is glued in. Again the pictures are obtained from a rational map encoding this subdivision. They are from joint work with Mario Bonk.

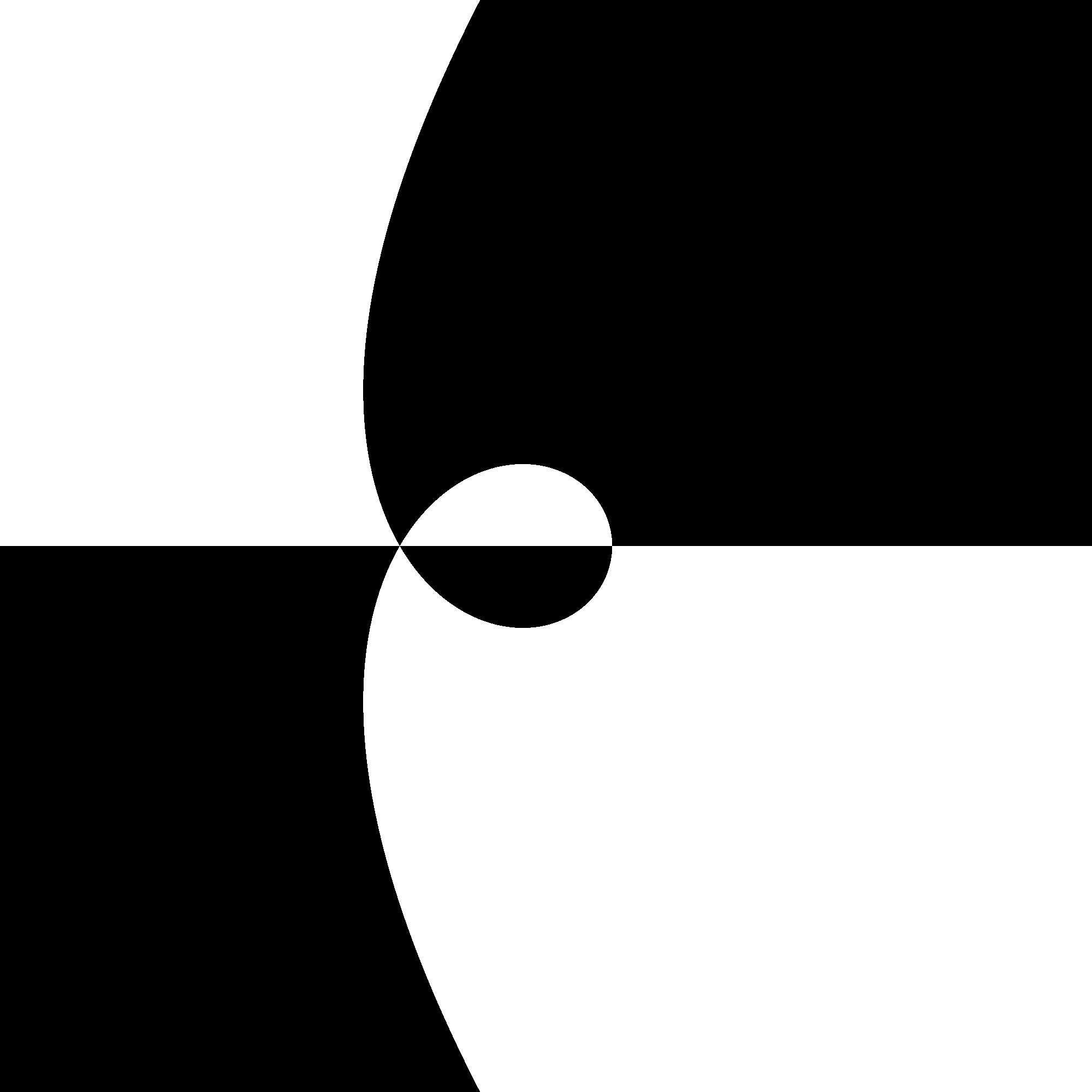
Snowspheres

Conformal tilings can be used to construct quasisymmetric parametrizations of self-similar surfaces. They are constructed analogous to the snowflake curve. More precisely the first five pictures are from a "snowball" constructed as follows. Start with a tetrahedron. Each face is divided into four equilateral triangles of half the side-length. Put a tetrahedron on the middle triangle. We obtain a polyhedral surface built from 4*6=24 small triangles. The procedure is iterated, meaning each small triangle is divided into four, and a tetrahedron is put on the middle triangle. The latter four pictures are obtained from a similar snowball. The construction is the same as before, except that we put an octahedron on the middle triangle in each step.

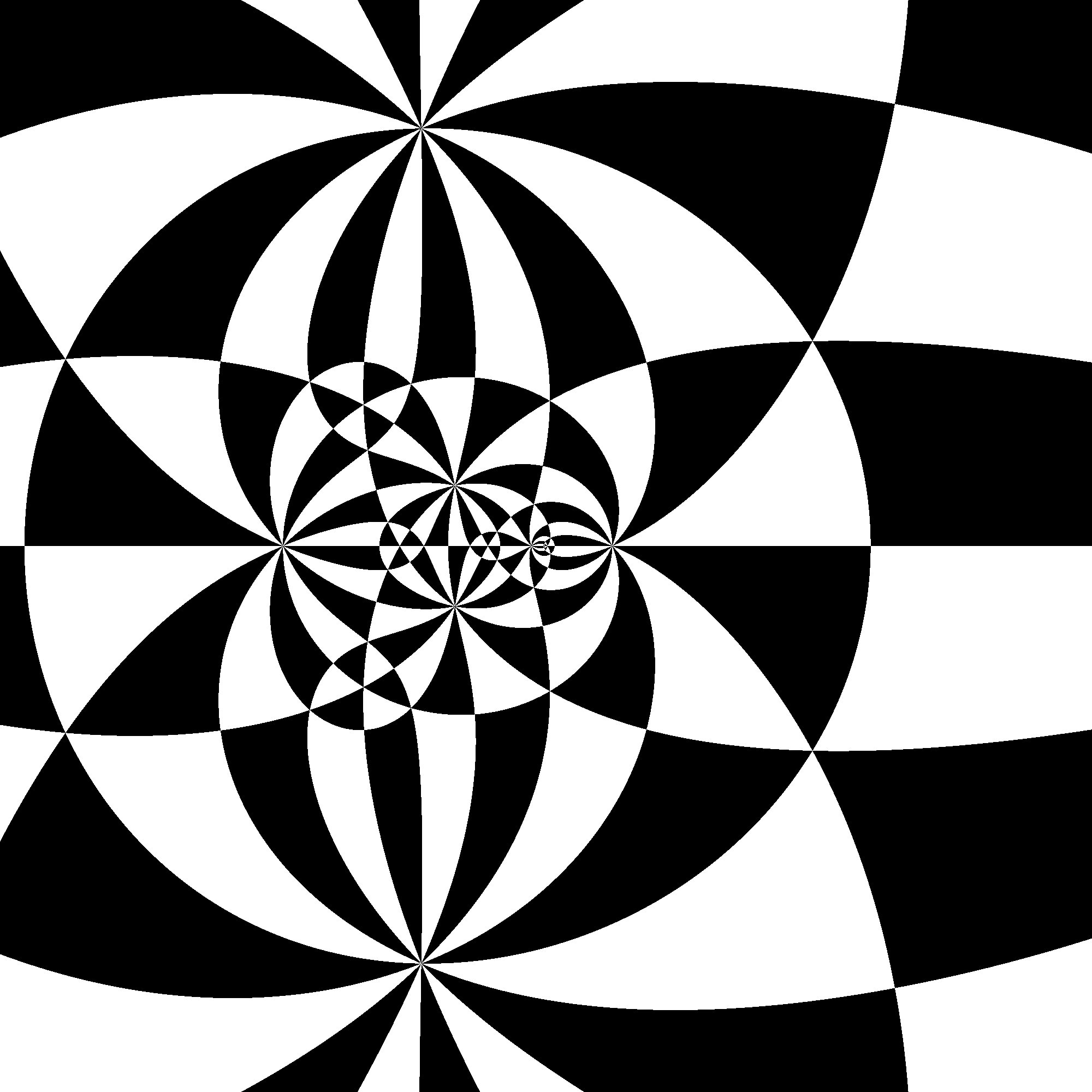
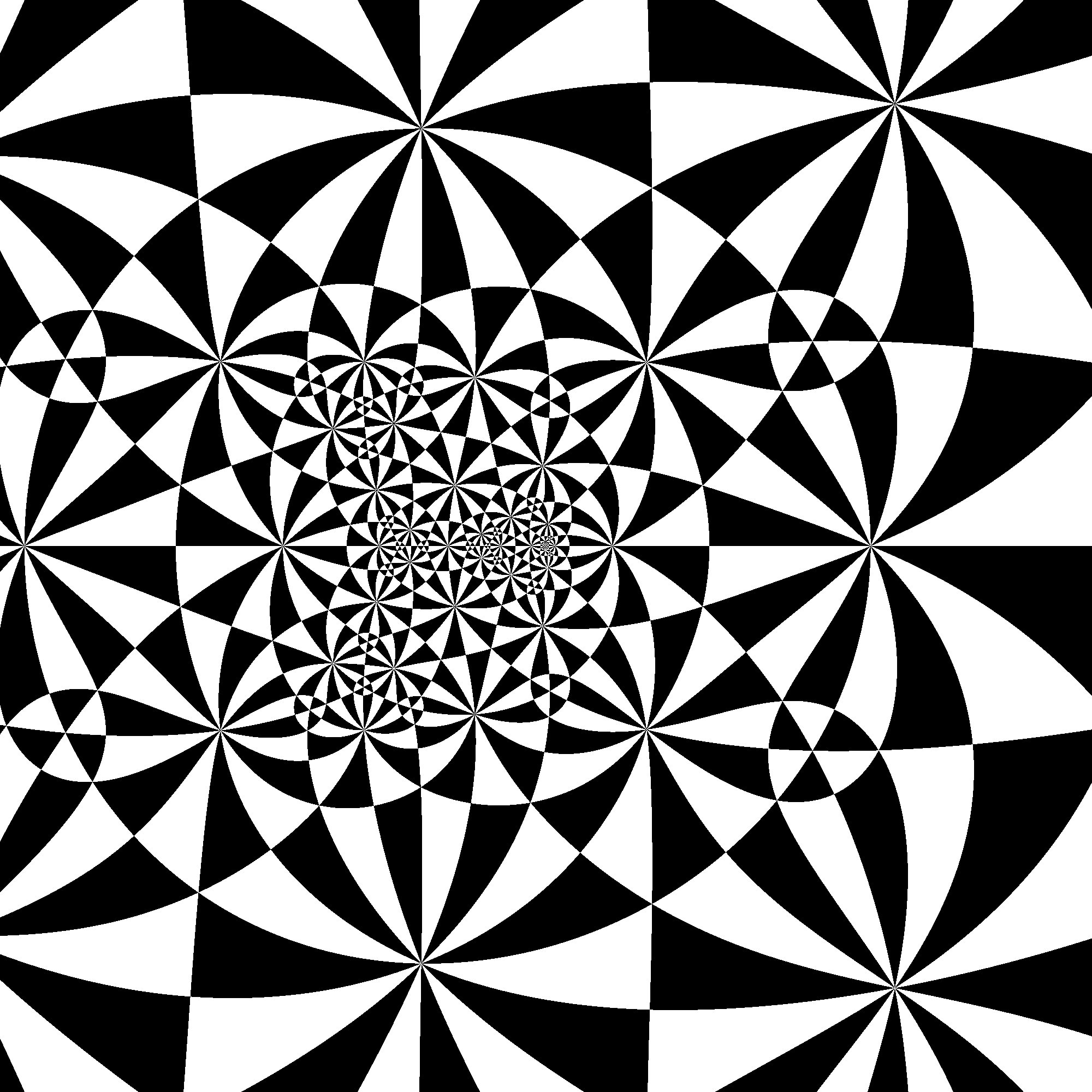
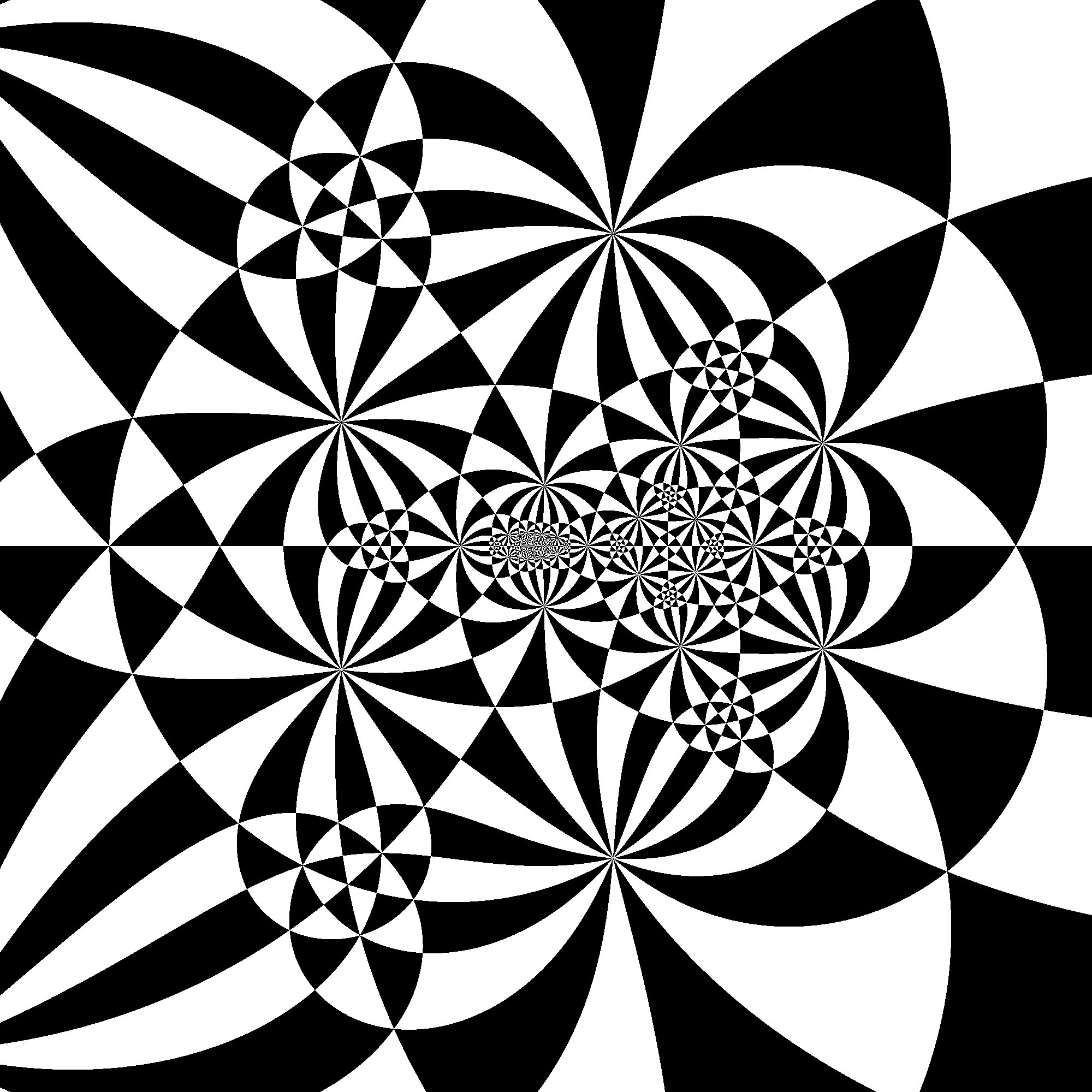
Another example