Bifid / binary / bifurcating / bipartite
All these adjectives are used in conjunction with the nouns 'tree' or 'stemma', they derive from Latin and contain the element bi- ‘two’. Bifid is derived from Latin bifidus ‘divided into two parts’, bipartitus is a Latin synonym for bifidus, and binarius means anything ‘that contains or consists of two’. 'To bifurcate' stems from Latin bifurcus ‘having two prongs or points’ (all Latin meanings from Lewis and Short 1879).
A bifid stemma is a stemma codicum in which the original or the archetype produces exactly two branches out of which the entire transmission ensues. The term was first used by Joseph Bédier who observed that, in the field of Old French manuscript traditions, almost all stemmata were 'bifid' which led him to question the validity of the Lachmannian approach (cf. Bédier 1913 and 1928). Bédier speaks of a silva portentosa (an 'unnatural / monstrous forest') of nearly exclusively bifid trees he found in the many stemmata of Old French texts he inspected. Several theories have been proposed to explain or rationalise this phenomenon (already by Bédier himself): partly based on alleged forms of mediaeval text transmission, partly on psychological grounds: according to the latter editors tend to continue trying to find Bindefehler until they end up with only two families (possibly mistaking some shared, but polygenetic innovations as Bindefehler). This has the convenient side-effect for the editor that he must (and: may) choose between the two family’s divergent reading instead of following the automatic criterion of choosing the reading of the majority of the families in most cases (unless all three groups differ, or in the case of more than three groups there be the same number exhibiting the most common readings). The psychological argument thus amounts to the fact that the editor would like to have some freedom in determining his text.
A glance at the many (and often complicated) stemmata printed in volume I of the Geschichte der Textüberlieferung seems to indicate that bifid stemmata are much less prevalent for classical (Greek and Latin) texts; this impression is confirmed looking at some mediaeval Latin editions printed in the collection Corpus Christianorum Continuatio Mediaevalis. Whether these differences are due to the much more standardised classical languages or to different approaches by the editors would be interesting to study. In a recent study of stemmata in Old Norse philology, Haugen (2015) came to very similar figures for bifid stemmata as in the Old French tradition, even if there has been very little contact between the critics of the two traditions. This is consistent with a methodological rather than a historical explanation, i.e. that the high incidence of bifid stemmata may reflect the procedure of building stemmata rather than the actual manuscript tradition.
In contrast a binary stemma or tree is one that has exclusively vertices (nodes) with at most two children (not only on the top level like bifid stemmata). Although it is rather unlikely that real traditions of any size are of this kind, one does meet such stemmata not too rarely (cf. examples printed in Bédier 1928). Again, this may be accounted for with a psychological argument as described above. In mathematics one speaks similarly of ternary and n-ary trees which have a maximum of 3 (or n) children anywhere. Bifurcating is a synonym for binary in manuscript studies, whereas bipartite may be used as a synonym for bifid or binary.
Illustration
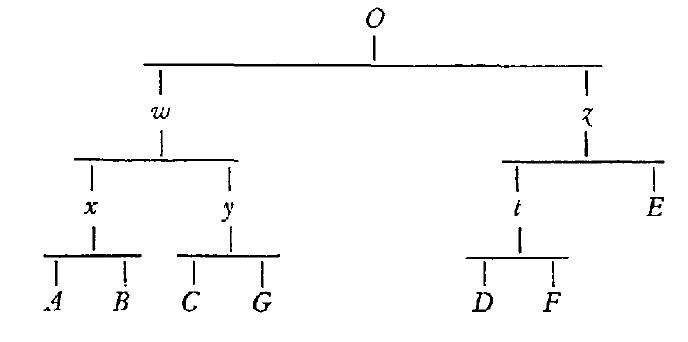
Fig. 1: The initially proposed binary stemma for the Lai de l'ombre by Bédier (reprinted in 1970, 6). Later Bédier accepted the critcism by Gaston Paris (1890) and modified the stemma to make it tripartite (by moving E directly below the archetype). According to Trovato, there is extra-stemmatic contamination in this tradition in the case of manuscript E (Trovato 2013, 294).
Other usage
In mathematics a bipartite graph is a graph whose nodes can be arranged into two disjoint sets such that every edge connects a node in one of them to one in the other (i.e. in either of the two sets there are no nodes that are connected with one another). It can be proved by induction that every tree is a bipartite graph (in this mathematical sense). These two meanings of ‘bipartite’ should therefore not be confused.
References
– Bédier, Joseph, ed. 1913. Jean Renart: Le lai de l’Ombre. Société des anciens textes français. Paris: Firmin-Didot.
– Bédier, Joseph. 1928. “La tradition manuscrite du Lai de l’Ombre: Réflexions sur l’art d’éditer les anciens textes.” Romania 54: 161–196, 321–356. – Reprint, Paris: Champion, 1970 gallica.bnf.fr/ark:/12148/bpt6k8980
– Castellani, Arrigo. 1957. Bédier avait-il raison? La méthode de Lachmann dans les éditions de textes du moyen age. Leçon inaugurale donnée à l’université de Fribourg le 2 juin 1954. Fribourg: Éditions universitaires.
– Guidi, Vincenzo, and Trovato, Paolo. 2004. “Sugli stemmi bipartiti: Decimazione, asimmetria e calcolo delle probabilità.” Filologia italiana 1: 9–48.
– Haugen, Odd Einar. 2015. “The silva portentosa of stemmatology: Bifurcation in the recension of Old Norse manuscripts.” Digital Scholarship in the Humanities. Advance access 21 march 2015, http://dx.doi.org/10.1093/llc/fqv002– Hunger, Herbert et al. 1961–1964. Geschichte der Textüberlieferung der antiken und mittelalterlichen Literatur. 2 vols. Zürich: Atlantis-Verlag.
– Lewis, Charlton, and Charles Short. 1879. A Latin Dictionary. London and New York: Harper and Brothers and Oxford University Press.
– Paris, Gaston. 1890. Review of Le Lai d'Ombre of Joseph Bédier. Romania 19: 609–615.
– Trovato, Paolo. 2013. “La tradizione manoscritta del ‘Lai de l’ombre’: Riflessioni sulle tecniche d’edizione primonovecentesche.” Romania 131: 338–380.
In other languages
DE: zweigespalten (Maas), zweispaltig, binär / zweigliedrig, Bifurkations- (only as first part of compounds), bipartit / zweiteilig (the usage is not fixed!)
FR: bifide / binaire / bifurqué / bipartite (usage as in English)
IT: bifido / binario / biforcato / bipartito.